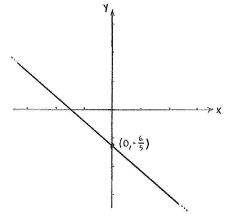Lösung 2.2:6c
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
The point of intersection is that point which satisfies the equations of both lines | The point of intersection is that point which satisfies the equations of both lines | ||
| - | {{ | + | {{Abgesetzte Formel||<math>4x+5y+6=0\qquad\text{and}\qquad x=0\,\textrm{.}</math>}} |
Substituting <math>x=0</math> into <math>4x+5y+6=0</math> gives | Substituting <math>x=0</math> into <math>4x+5y+6=0</math> gives | ||
| - | {{ | + | {{Abgesetzte Formel||<math>4\cdot 0+5y+6=0\quad\Leftrightarrow\quad y=-\frac{6}{5}\,\textrm{.}</math>}} |
This gives the point of intersection as <math>\bigl(0,-\tfrac{6}{5}\bigr)</math>. | This gives the point of intersection as <math>\bigl(0,-\tfrac{6}{5}\bigr)</math>. | ||
Version vom 08:29, 22. Okt. 2008
The point of intersection is that point which satisfies the equations of both lines
| \displaystyle 4x+5y+6=0\qquad\text{and}\qquad x=0\,\textrm{.} |
Substituting \displaystyle x=0 into \displaystyle 4x+5y+6=0 gives
| \displaystyle 4\cdot 0+5y+6=0\quad\Leftrightarrow\quad y=-\frac{6}{5}\,\textrm{.} |
This gives the point of intersection as \displaystyle \bigl(0,-\tfrac{6}{5}\bigr).