Lösung 4.1:5a

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 4.1:5a moved to Solution 4.1:5a: Robot: moved page)
Zeile 2: Zeile 2:
[[Image:4_1_5_a.gif|center]]
[[Image:4_1_5_a.gif|center]]
-
<center> [[Image:4_1_5a.gif]] </center>
 
{{NAVCONTENT_STOP}}
{{NAVCONTENT_STOP}}
 +
 +
 +
A circle is defined as all the points which have a fixed distance to the circle's midpoint. Hence, a point
 +
<math>\left( x \right.,\left. y \right)</math>
 +
lies on our circle if and only if its distance to the point
 +
<math>\left( 1 \right.,\left. 3 \right)</math>
 +
is exactly
 +
<math>2</math>. Using the distance formula, we can express this condition as
 +
 +
 +
<math>\sqrt{\left( x-1 \right)^{2}+\left( y-2 \right)^{2}}=2</math>
 +
 +
 +
After squaring, we obtain the equation of the circle in standard form:
 +
 +
 +
<math>\left( x-1 \right)^{2}+\left( y-2 \right)^{2}=4</math>

Version vom 11:09, 27. Sep. 2008


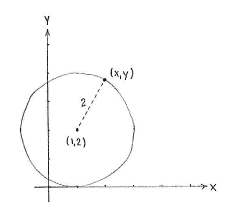
A circle is defined as all the points which have a fixed distance to the circle's midpoint. Hence, a point \displaystyle \left( x \right.,\left. y \right) lies on our circle if and only if its distance to the point \displaystyle \left( 1 \right.,\left. 3 \right) is exactly \displaystyle 2. Using the distance formula, we can express this condition as


\displaystyle \sqrt{\left( x-1 \right)^{2}+\left( y-2 \right)^{2}}=2


After squaring, we obtain the equation of the circle in standard form:


\displaystyle \left( x-1 \right)^{2}+\left( y-2 \right)^{2}=4

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.1:5a“