Solution 4.3:3d
From Förberedande kurs i matematik 1
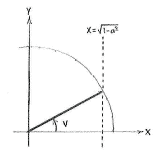
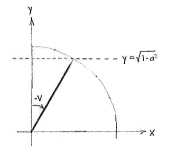
The expression for the angle \displaystyle \pi/2 - v differs from \displaystyle \pi/2 by as much as \displaystyle -v differs from \displaystyle 0. This means that \displaystyle \pi/2-v makes the same angle with the positive y-axis as \displaystyle -v makes with the positive x-axis.
| 
| |
| Angle v | Angle π/2 - v |
Therefore, the angle \displaystyle \pi/2 - v has a y-coordinate which is equal to the x-coordinate for the angle v, i.e.
| \displaystyle \sin\Bigl(\frac{\pi}{2} - v\Bigr) = \cos v |
and from exercise c, we know that \displaystyle \cos v = \sqrt{1-a^2}\,,
| \displaystyle \sin\Bigl(\frac{\pi}{2}-v\Bigr) = \sqrt{1-a^2}\,\textrm{.} |
