Lösung 4.4:5b
Aus Online Mathematik Brückenkurs 1
Let's first investigate when the equality
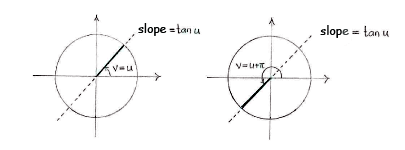
is satisfied. Because \displaystyle \tan u can be interpreted as the slope (gradient) of the line which makes an angle u with the positive x-axis, we see that for a fixed value of \displaystyle \tan u, there are two angles v in the unit circle with this slope,
The angle v has the same slope after every half turn, so if we add multiples of \displaystyle \pi to u, we will obtain all the angles v which satisfy the equality
where n is an arbitrary integer.
If we apply this result to the equation
we see that the solutions are given by
and solving for x gives