Aus Online Mathematik Brückenkurs 1

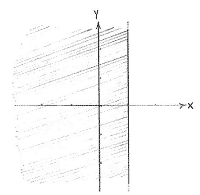
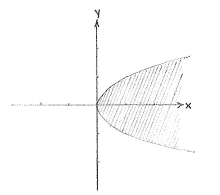
The expression \displaystyle 1\ge x\ge y^{2} means that we have a region which is defined by the two inequalities \displaystyle 1\ge x and \displaystyle x\ge y^{2}. The first inequality gives us the region to the left of the line \displaystyle x=1. If the other inequality had been instead \displaystyle y\ge x^{2}, we would have a region above the parabola \displaystyle y=x^{2}, but in our case x and y have reversed roles, so the inequality \displaystyle x\ge y^{2} defines the same type of parabolic region, but with the x- and y-axes having changed place.

The region 1 ≥ x		The region x ≥ y²

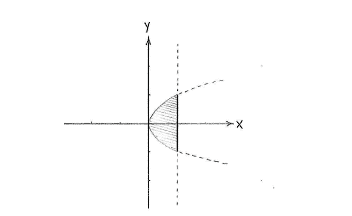
Together, the inequalities define the region that is bordered on the left by the parabola and on the right by the line.

The region 1 ≥ x ≥ y²