Aus Online Mathematik Brückenkurs 1

The points of intersection are those points on the curve which also lie on the \displaystyle ~x -axis, i.e. they are those points which satisfy both the equation of the curve \displaystyle y=x^{\text{2}}-\text{5}x+\text{6} and the equation of the \displaystyle ~x -axis \displaystyle y=0,

\displaystyle \left\{ \begin{matrix} y=x^{\text{2}}-\text{5}x+\text{6} \\ y=0\quad \quad \quad \quad \\ \end{matrix} \right.

This system of equations gives directly that \displaystyle y=0 and that \displaystyle ~x must satisfy the second-order equation \displaystyle x^{\text{2}}-\text{5}x+\text{6}=0 . By completing the square, we obtain that the left-hand side is

\displaystyle \begin{align} & x^{\text{2}}-\text{5}x+\text{6}=\left( x-\frac{5}{2} \right)^{2}-\left( \frac{5}{2} \right)^{2}+6 \\ & =\left( x-\frac{5}{2} \right)^{2}-\frac{25}{4}+\frac{24}{4}=\left( x-\frac{5}{2} \right)^{2}-\frac{1}{4} \\ \end{align}

and this gives that the equation has solutions

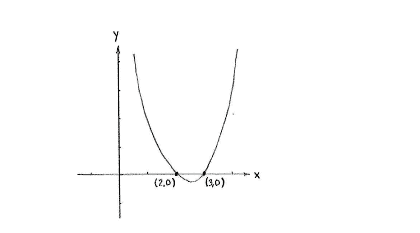
\displaystyle x=\frac{5}{2}\pm \frac{1}{2}, i.e. \displaystyle x=\frac{5}{2}-\frac{1}{2}=\frac{4}{2}=2 and \displaystyle x=\frac{5}{2}+\frac{1}{2}=\frac{6}{2}=3.

The intersection points are therefore \displaystyle \left( 2 \right.,\left. 0 \right) and \displaystyle \left( 3 \right.,\left. 0 \right).