Aus Online Mathematik Brückenkurs 1

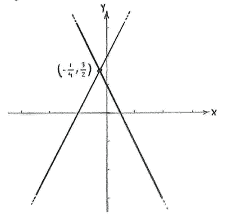
The lines have a point of intersection at that point which simultaneously satisfies the equations of both lines:

\displaystyle 2x+y-1=0 and \displaystyle y-2x-2=0.

If we make \displaystyle y the subject of the second equation \displaystyle y-2x-2=0 and substitute it into the first equation, we obtain an equation which only contains \displaystyle x,

\displaystyle 2x+\left( 2x+2 \right)-1=0\ \Leftrightarrow \ 4x+1=0

which gives that \displaystyle x=-{1}/{4}\;. Then, from the relation \displaystyle y=2x+2, we obtain \displaystyle y=2\left( -{1}/{4}\; \right)+2={3}/{2}\;.

The point of intersection is \displaystyle \left( -\frac{1}{4} \right.,\left. \frac{3}{2} \right).

We check for safety's sake that \displaystyle \left( -\frac{1}{4} \right.,\left. \frac{3}{2} \right) really satisfies both equations:

We check for safety's sake that \displaystyle \left( -\frac{1}{4} \right.,\left. \frac{3}{2} \right) really satisfies both equations:

\displaystyle 2x+y-1=0: LHS = \displaystyle 2\left( -\frac{1}{4} \right)+\frac{3}{2}-1=-\frac{1}{2}+\frac{3}{2}-\frac{2}{2}=0 =RHS

\displaystyle y-2x-2=0: LHS = \displaystyle \frac{3}{2}-2\left( -\frac{1}{4} \right)-2=\frac{3}{2}+\frac{1}{2}-\frac{4}{2}=0 =RHS