Aus Online Mathematik Brückenkurs 1

The right-hand side of the equation is a constant, so the equation is in fact a normal trigonometric equation of the type \displaystyle \text{cos }x=a.

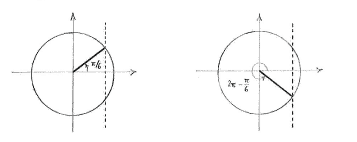
In this case, we can see directly that one solution is \displaystyle x={\pi }/{6}\;. Using the unit circle, it follows that \displaystyle x=2\pi -{\pi }/{6}\;={11\pi }/{6}\; is the only other solution between \displaystyle 0 and \displaystyle \text{2}\pi .

We obtain all solutions to the equation if we add multiples of \displaystyle \text{2}\pi to the two solutions above:

\displaystyle x=\frac{\pi }{6}+2n\pi and \displaystyle x=\frac{11\pi }{6}+2n\pi

where \displaystyle n is an arbitrary integer.