Aus Online Mathematik Brückenkurs 1

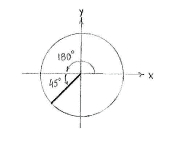
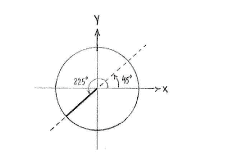
If we draw the angle \displaystyle \text{225}^{\circ }\text{ }=\text{ 18}0^{\circ }\text{ }+\text{ 45}^{\circ } on a unit circle, we see that it makes an angle of \displaystyle \text{45}^{\circ } with the negative \displaystyle x -axis.

This means that \displaystyle \text{tan 225}^{\circ }, which is the gradient of the line that makes an angle of \displaystyle \text{45}^{\circ } with the positive \displaystyle x -axis, equals \displaystyle \text{tan 225}^{\circ }, because the line which makes an angle of \displaystyle \text{45}^{\circ } has the same slope:

\displaystyle \tan 225^{\circ }\text{ }=\tan \text{45}^{\circ }=\frac{\sin \text{45}^{\circ }}{\cos \text{45}^{\circ }}=\frac{\frac{1}{\sqrt{2}}}{\frac{1}{\sqrt{2}}}=1