Lösung 4.2:4e
Aus Online Mathematik Brückenkurs 1
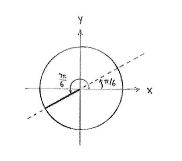
If we write the angle \displaystyle \frac{7\pi }{6} as
\displaystyle \frac{7\pi }{6}=\frac{6\pi +\pi }{6}=\pi +\frac{\pi }{6}
we see that the angle \displaystyle \frac{7\pi }{6} on a unit circle is in the third quadrant and makes an angle \displaystyle \frac{\pi }{6} with the negative \displaystyle x -axis.
Geometrically, \displaystyle \tan \frac{7\pi }{6} is defined as the gradient of the line having an angle \displaystyle \frac{7\pi }{6} and, because this line has the same slope as the line having angle \displaystyle \frac{\pi }{6}, we have that
\displaystyle \tan \frac{7\pi }{6}=\tan \frac{\pi }{6}=\frac{\sin \frac{\pi }{6}}{\cos \frac{\pi }{6}}=\frac{\frac{1}{2}}{\frac{\sqrt{3}}{2}}=\frac{1}{\sqrt{3}}