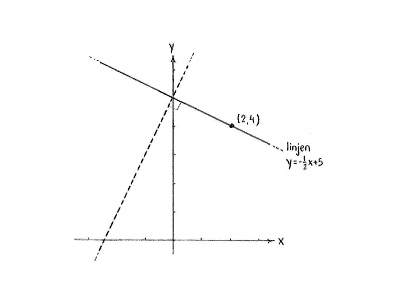
Lösung 2.2:5d
Aus Online Mathematik Brückenkurs 1
If two non-vertical lines are perpendicular to each other, their gradients \displaystyle k_{1} and \displaystyle k_{2} satisfy the relation
\displaystyle k_{1}k_{2}=-1, and from this we have that the line we are looking for must have a gradient that is given by
\displaystyle k_{2}=-\frac{1}{k_{1}}=-\frac{1}{2}
since the line
\displaystyle y=2x+5
has a gradient
\displaystyle k_{1}=2
(the coefficient in front of
\displaystyle x
).
The line we are looking for can thus be written in the form
\displaystyle y=-\frac{1}{2}x+m
with
\displaystyle m
as an unknown constant.
Because the point \displaystyle \left( 2 \right.,\left. 4 \right) should lie on the line, \displaystyle \left( 2 \right.,\left. 4 \right) must satisfy the equation of the line,
\displaystyle 4=-\frac{1}{2}\centerdot 2+m
i.e.
\displaystyle m=5. The equation of the line is .
\displaystyle y=-\frac{1}{2}x+5