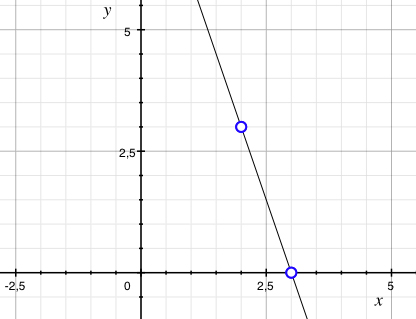
Lösung 2.2:5a
Aus Online Mathematik Brückenkurs 1
Let's write down the equation for a straight line as
\displaystyle y=kx+m
where
\displaystyle k
and
\displaystyle m
are constants which we shall determine.
Since the points \displaystyle \left( 2 \right., \left. 3 \right) and \displaystyle \left( 3 \right., \left. 0 \right) should lie on the line, they must also satisfy the equation of the line,
\displaystyle 3=k\centerdot 2+m
and
\displaystyle 0=k\centerdot 3+m
If we take the difference between the equations,
\displaystyle m
disappears and we can work out the gradient
\displaystyle k,
\displaystyle 3-0=k\centerdot 2+m-\left( k\centerdot 3+m \right)
\displaystyle 3=-k
Substituting this into the equation \displaystyle 0=k\centerdot 3+m then gives us a value for \displaystyle m,
\displaystyle m=-3k=-3\centerdot \left( -3 \right)=9
The equation of the line is thus
\displaystyle y=-3x+9.
NOTE: To be completely certain that we have calculated correctly, we check that the points \displaystyle \left( 2 \right., \left. 3 \right) and \displaystyle \left( 3 \right., \left. 0 \right) satisfy the equation of the line:
\displaystyle \left( x \right., \left. y \right)=\left( 2 \right., \left. 3 \right): LHS= \displaystyle 3 and RHS= \displaystyle -3\centerdot 2+9=3
\displaystyle \left( x \right., \left. y \right)=\left( 3 \right., \left. 0 \right): LHS=
\displaystyle 0
and LHS=
\displaystyle -3\centerdot 3+9=0