Aus Online Mathematik Brückenkurs 1

Wir finden zuerst die allgemeine Lösung der Gleichung, und sehen danach welche der Winkeln im Intervall zwischen \displaystyle 0^{\circ} und \displaystyle 360^{\circ}\, liegen.

Wir betrachten zuerst den Ausdruck \displaystyle 2v+10^{\circ}, und erhalten die Lösung

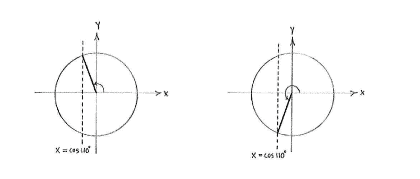
\displaystyle 2v + 10^{\circ} = 110^{\circ}\,\textrm{.}

Es gibt noch eine Lösung im Einheitskreis, nämlich im dritten Quadrant, mit denselben Winkel zur negativen y-Achse, wie der Winkel \displaystyle 110^{\circ} zur positiven y-Achse hat, nur mit anderen Vorzeichen. Daher erhalten wir die zweite Lösung

\displaystyle 2v + 10^{\circ} = 270^{\circ} - 20^{\circ} = 250^{\circ}\,\textrm{.}

und die allgemeine Lösung ist

\displaystyle \left\{\begin{align} 2v + 10^{\circ} &= 110^{\circ} + n\cdot 360^{\circ}\quad\text{und}\\[5pt] 2v + 10^{\circ} &= 250^{\circ} + n\cdot 360^{\circ}\,,\end{align}\right.

Lösen wir w erhalten wir

\displaystyle \left\{\begin{align} v &= 50^{\circ} + n\cdot 180^{\circ}\quad\text{und}\\[5pt] v &= 120^{\circ} + n\cdot 180^{\circ}\end{align}\right.

für verschiedene n erhalten wir unter anderen die Lösungen

\displaystyle \cdots\cdots		\displaystyle \cdots\cdots		\displaystyle \cdots\cdots
\displaystyle n=-2:		\displaystyle v = 50^{\circ} - 2\cdot 180^{\circ} = -310^{\circ}		\displaystyle v = 120^{\circ } - 2\cdot 180^{\circ} = -240^{\circ}
\displaystyle n=-1:		\displaystyle v = 50^{\circ} - 1\cdot 180^{\circ} = -130^{\circ}		\displaystyle v = 120^{\circ} - 1\cdot 180^{\circ} = -60^{\circ}
\displaystyle n=0:		\displaystyle v = 50^{\circ} + 0\cdot 180^{\circ} = 50^{\circ}		\displaystyle v = 120^{\circ} + 0\cdot 180^{\circ} = 120^{\circ}
\displaystyle n=1:		\displaystyle v = 50^{\circ} + 1\cdot 180^{\circ} = 230^{\circ}		\displaystyle v = 120^{\circ} + 1\cdot 180^{\circ} = 300^{\circ}
\displaystyle n=2:		\displaystyle v = 50^{\circ} + 2\cdot 180^{\circ} = 410^{\circ}		\displaystyle v = 120^{\circ} + 2\cdot 180^{\circ} = 480^{\circ}
\displaystyle n=3:		\displaystyle v = 50^{\circ} + 3\cdot 180^{\circ} = 590^{\circ}		\displaystyle v = 120^{\circ} + 3\cdot 180^{\circ} = 660^{\circ}
\displaystyle \cdots\cdots		\displaystyle \cdots\cdots		\displaystyle \cdots\cdots

Hieraus sehen wir die Lösungen die im Intervall von \displaystyle 0^{\circ} bis \displaystyle 360^{\circ} sind:

\displaystyle v = 50^{\circ},\quad v=120^{\circ },\quad v=230^{\circ}\quad\text{and}\quad v=300^{\circ}\,\textrm{.}