Aus Online Mathematik Brückenkurs 1

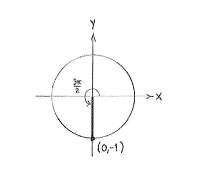
Wir subtrahieren \displaystyle 2\pi vom Winkel math>{7\pi }/{2}\,</math>, so oft bis wir einen Winkel zwischen \displaystyle 0 und \displaystyle 2\pi erhalten,

\displaystyle \cos\frac{7\pi}{2} = \cos\Bigl(\frac{7\pi}{2}-2\pi\Bigr) = \cos\frac{3\pi}{2}\,\textrm{.}

Wir sehen dass die Gerade mit den Winkel \displaystyle 3\pi/2 zur x-Achse den Einheitskreis im Punkt (0,-1) schneidet. Die x-Koordinate des Schnittpunktes ist also \displaystyle 0 und daher ist \displaystyle \cos (7\pi/2) = \cos (3\pi/2) = 0\,.