Lösung 4.3:9
Aus Online Mathematik Brückenkurs 1
Using the formula for double angles on sin \displaystyle 160^{\circ } gives
\displaystyle \sin 160^{\circ }=2\cos 80^{\circ }\sin 80^{\circ }
On the right-hand side, we see that the factor
\displaystyle \cos 80^{\circ }
has appeared, and if we use the formula for double angles on the second factor (
\displaystyle \sin 80^{\circ }
),
\displaystyle 2\cos 80^{\circ }\sin 80^{\circ }=2\cos 80^{\circ }\centerdot 2\cos 40^{\circ }\sin 40^{\circ }
we obtain a further factor
\displaystyle \cos 40^{\circ }. A final application of the formula for double angles on
\displaystyle \sin 40^{\circ }
gives us all three cosine factors:
\displaystyle 2\cos 80^{\circ }\centerdot 2\cos 40^{\circ }\centerdot \sin 40^{\circ }=2\cos 80^{\circ }\centerdot 2\cos 40^{\circ }\centerdot 2\cos 20^{\circ }\sin 20^{\circ }
We have thus succeeded in showing that
\displaystyle \sin 160^{\circ }=8\cos 80^{\circ }\centerdot \cos 40^{\circ }\centerdot \cos 20^{\circ }\sin 20^{\circ }
which can also be written as
\displaystyle \cos 80^{\circ }\centerdot \cos 40^{\circ }\centerdot \cos 20^{\circ }=\frac{\sin 160^{\circ }}{8\sin 20^{\circ }}
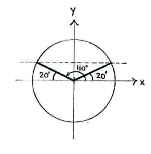
If we draw the unit circle, we see that
\displaystyle 160^{\circ }
makes an angle of
\displaystyle 20^{\circ }
with the negative
\displaystyle x
-axis, and therefore the angles
\displaystyle 20^{\circ }
and
\displaystyle 160^{\circ }
have the same
\displaystyle y
-coordinate in the unit circle, i.e.
\displaystyle \sin 20^{\circ }=\sin 160^{\circ }.
This shows that
\displaystyle \cos 80^{\circ }\centerdot \cos 40^{\circ }\centerdot \cos 20^{\circ }=\frac{\sin 160^{\circ }}{8\sin 20^{\circ }}=\frac{1}{8}