Lösung 4.2:5a
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
| Zeile 6: | Zeile 6: | ||
{| width="100%" | {| width="100%" | ||
| - | |width="50%" align="center"|[[Image: | + | |width="50%" align="center"|[[Image:4_2_5_a2_de.gif|center]] |
|width="50%" align="left"|<math>\begin{align}\text{Gegenkathete} &= 1\cdot\sin 45^{\circ} = \dfrac{1}{\sqrt{2}}\\[5pt] \text{Ankathete} &= 1\cdot\cos 45^{\circ} = \frac{1}{\sqrt{2}}\end{align}</math> | |width="50%" align="left"|<math>\begin{align}\text{Gegenkathete} &= 1\cdot\sin 45^{\circ} = \dfrac{1}{\sqrt{2}}\\[5pt] \text{Ankathete} &= 1\cdot\cos 45^{\circ} = \frac{1}{\sqrt{2}}\end{align}</math> | ||
|} | |} | ||
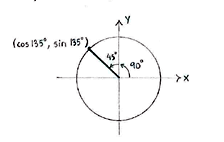
Der Punkt hat also die Koordinaten <math>( -1/\!\sqrt{2}, 1/\!\sqrt{2})</math>. Daher ist <math>\cos 135^{\circ} = -1/\!\sqrt{2}\,</math>. | Der Punkt hat also die Koordinaten <math>( -1/\!\sqrt{2}, 1/\!\sqrt{2})</math>. Daher ist <math>\cos 135^{\circ} = -1/\!\sqrt{2}\,</math>. | ||
Version vom 15:48, 30. Jul. 2009
Da der Winkel \displaystyle 135^{\circ} = 90^{\circ} + 45^{\circ}, \displaystyle 135^{\circ} im zweiten Quadranten liegt, bildet er den Winkel \displaystyle 45^{\circ} mit der positiven y-Achse
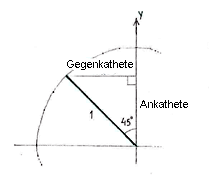
Wir betrachten das Dreieck im zweiten Quadrant wie im Bild, um die Koordinaten des Punktes am Einheitskreis, der den Winkel \displaystyle 135^{\circ} entspricht, zu bestimmen.
| \displaystyle \begin{align}\text{Gegenkathete} &= 1\cdot\sin 45^{\circ} = \dfrac{1}{\sqrt{2}}\\[5pt] \text{Ankathete} &= 1\cdot\cos 45^{\circ} = \frac{1}{\sqrt{2}}\end{align} |
Der Punkt hat also die Koordinaten \displaystyle ( -1/\!\sqrt{2}, 1/\!\sqrt{2}). Daher ist \displaystyle \cos 135^{\circ} = -1/\!\sqrt{2}\,.