Lösung 4.4:5c

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (hat „Solution 4.4:5c“ nach „Lösung 4.4:5c“ verschoben: Robot: moved page)
Zeile 1: Zeile 1:
-
For a fixed value of ''u'', an equality of the form
+
Für einen Konstanten ''u'', ist die Gleichung
{{Abgesetzte Formel||<math>\cos u=\cos v</math>}}
{{Abgesetzte Formel||<math>\cos u=\cos v</math>}}
-
is satisfied by two angles ''v'' in the unit circle,
+
für folgende Winkeln ''v'' erfüllt
-
{{Abgesetzte Formel||<math>v=u\qquad\text{and}\qquad v=-u\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>v=u\qquad\text{und}\qquad v=-u\,\textrm{.}</math>}}
[[Image:4_4_5_c.gif|center]]
[[Image:4_4_5_c.gif|center]]
-
This means that all angles ''v'' which satisfy the equality are
+
Also sind die allgemeinen Lösungen
-
{{Abgesetzte Formel||<math>v=u+2n\pi\qquad\text{and}\qquad v=-u+2n\pi\,,</math>}}
+
{{Abgesetzte Formel||<math>v=u+2n\pi\qquad\text{und}\qquad v=-u+2n\pi\,,</math>}}
-
where ''n'' is an arbitrary integer.
+
Also hat die Gleichung
-
 
+
-
Therefore, the equation
+
{{Abgesetzte Formel||<math>\cos 5x=\cos (x+\pi/5)</math>}}
{{Abgesetzte Formel||<math>\cos 5x=\cos (x+\pi/5)</math>}}
-
has the solutions
+
die Lösungen
-
{{Abgesetzte Formel||<math>\left\{\begin{align} 5x&=x+\frac{\pi}{5}+2n\pi\quad\text{or}\\[5pt] 5x &= -x-\frac{\pi}{5}+2n\pi\,\textrm{.}\end{align}\right.</math>}}
+
{{Abgesetzte Formel||<math>\left\{\begin{align} 5x&=x+\frac{\pi}{5}+2n\pi\quad\text{oder}\\[5pt] 5x &= -x-\frac{\pi}{5}+2n\pi\,\textrm{.}\end{align}\right.</math>}}
-
If we collect ''x'' onto one side, we end up with
+
Lösen wir die Gleichung für ''x'', erhalten wir
{{Abgesetzte Formel||<math>\left\{\begin{align}
{{Abgesetzte Formel||<math>\left\{\begin{align}
Zeile 29: Zeile 27:
x &= -\frac{\pi }{30}+\frac{n\pi}{3}\,,
x &= -\frac{\pi }{30}+\frac{n\pi}{3}\,,
\end{align}\right.</math>}}
\end{align}\right.</math>}}
- 
-
where ''n'' is an arbitrary integer.
 

Version vom 11:21, 7. Apr. 2009

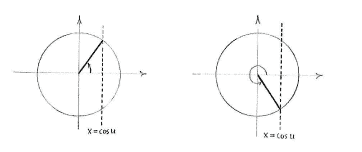
Für einen Konstanten u, ist die Gleichung

\displaystyle \cos u=\cos v

für folgende Winkeln v erfüllt

\displaystyle v=u\qquad\text{und}\qquad v=-u\,\textrm{.}

Also sind die allgemeinen Lösungen

\displaystyle v=u+2n\pi\qquad\text{und}\qquad v=-u+2n\pi\,,

Also hat die Gleichung

\displaystyle \cos 5x=\cos (x+\pi/5)

die Lösungen

\displaystyle \left\{\begin{align} 5x&=x+\frac{\pi}{5}+2n\pi\quad\text{oder}\\[5pt] 5x &= -x-\frac{\pi}{5}+2n\pi\,\textrm{.}\end{align}\right.

Lösen wir die Gleichung für x, erhalten wir

\displaystyle \left\{\begin{align}

x &= \frac{\pi}{20} + \frac{n\pi}{2}\,,\\[5pt] x &= -\frac{\pi }{30}+\frac{n\pi}{3}\,, \end{align}\right.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.4:5c“