Lösung 4.2:5b
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (hat „Solution 4.2:5b“ nach „Lösung 4.2:5b“ verschoben: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
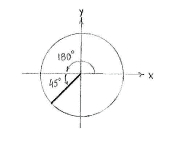
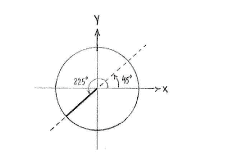
| - | + | Wir zeichnen den Winkel <math>225^{\circ} = 180^{\circ} + 45^{\circ}</math> am einheitskreis, und sehen dass er den Winkel <math>45^{\circ}</math> mit der negativen ''x''-Achse bildet. | |
[[Image:4_2_5_b1.gif|center]] | [[Image:4_2_5_b1.gif|center]] | ||
| - | + | Also ist <math>\tan 225^{\circ} = \tan 45^{\circ}</math>, nachdem die beiden Geraden mit diesen Winkeln dieselbe Steigung haben, | |
{{Abgesetzte Formel||<math>\tan 225^{\circ} = \tan 45^{\circ} = \frac{\sin 45^{\circ}}{\cos 45^{\circ}} = \frac{\dfrac{1}{\sqrt{2}}}{\dfrac{1}{\sqrt{2}}} = 1\,\textrm{.}</math>}} | {{Abgesetzte Formel||<math>\tan 225^{\circ} = \tan 45^{\circ} = \frac{\sin 45^{\circ}}{\cos 45^{\circ}} = \frac{\dfrac{1}{\sqrt{2}}}{\dfrac{1}{\sqrt{2}}} = 1\,\textrm{.}</math>}} | ||
[[Image:4_2_5_b2.gif|center]] | [[Image:4_2_5_b2.gif|center]] | ||
Version vom 16:01, 4. Apr. 2009
Wir zeichnen den Winkel \displaystyle 225^{\circ} = 180^{\circ} + 45^{\circ} am einheitskreis, und sehen dass er den Winkel \displaystyle 45^{\circ} mit der negativen x-Achse bildet.
Also ist \displaystyle \tan 225^{\circ} = \tan 45^{\circ}, nachdem die beiden Geraden mit diesen Winkeln dieselbe Steigung haben,
| \displaystyle \tan 225^{\circ} = \tan 45^{\circ} = \frac{\sin 45^{\circ}}{\cos 45^{\circ}} = \frac{\dfrac{1}{\sqrt{2}}}{\dfrac{1}{\sqrt{2}}} = 1\,\textrm{.} |