Lösung 4.1:7c
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (hat „Solution 4.1:7c“ nach „Lösung 4.1:7c“ verschoben: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | + | Wir benutxen quadratische Ergänzung | |
{{Abgesetzte Formel||<math>\begin{align} | {{Abgesetzte Formel||<math>\begin{align} | ||
| Zeile 6: | Zeile 6: | ||
\end{align}</math>}} | \end{align}</math>}} | ||
| - | + | und erhalten so die Gleichung | |
{{Abgesetzte Formel||<math>\begin{align} | {{Abgesetzte Formel||<math>\begin{align} | ||
| Zeile 13: | Zeile 13: | ||
\end{align}</math>}} | \end{align}</math>}} | ||
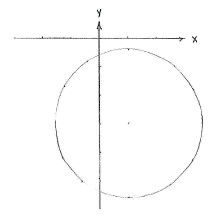
| - | + | Also hat der Kreis den Mittelpunkt (1,-3) und den Radius <math>\sqrt{7}\,</math>. | |
<center> [[Image:4_1_7_c.gif]] </center> | <center> [[Image:4_1_7_c.gif]] </center> | ||
Version vom 18:21, 2. Apr. 2009
Wir benutxen quadratische Ergänzung
| \displaystyle \begin{align}
x^2 - 2x &= (x-1)^2 - 1^2\,,\\[5pt] y^2 + 6y &= (y+3)^2 - 3^2\,, \end{align} |
und erhalten so die Gleichung
| \displaystyle \begin{align}
(x-1)^2 - 1 + (y+3)^2 - 9 &= -3\,,\\[5pt] (x-1)^2 + (y+3)^2 &= 7\,\textrm{.} \end{align} |
Also hat der Kreis den Mittelpunkt (1,-3) und den Radius \displaystyle \sqrt{7}\,.