Lösung 2.3:8c

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (hat „Solution 2.3:8c“ nach „Lösung 2.3:8c“ verschoben: Robot: moved page)
Zeile 1: Zeile 1:
-
By completing the square, we can rewrite the function as
+
Durch quadratische Ergänzung können wir den Ausdruck umschreiben
{{Abgesetzte Formel||<math>f(x) = x^{2}-6x+11 = (x-3)^{2} - 3^{2} + 11 = (x-3)^{2} + 2,</math>}}
{{Abgesetzte Formel||<math>f(x) = x^{2}-6x+11 = (x-3)^{2} - 3^{2} + 11 = (x-3)^{2} + 2,</math>}}
-
and when the function is written in this way, we see that the graph <math>y = (x-3)^{2} + 2</math> is the same curve as the parabola <math>y=x^{2}</math>, but shifted two units up and three units to the right (see sub-exercise a and b).
+
So sehen wir dass der Graph von der Funktion <math>y = (x-3)^{2} + 2</math> der Graph von der Funktion <math>y=x^{2}</math> ist, nur zwei Einheiten nach oben, und drei Einheiten nach rechts verschoben (Sehen Sie 8a und 8b).

Version vom 19:29, 16. Mär. 2009

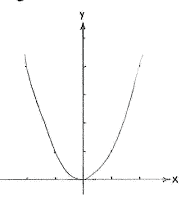
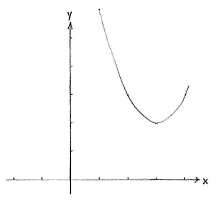
Durch quadratische Ergänzung können wir den Ausdruck umschreiben

\displaystyle f(x) = x^{2}-6x+11 = (x-3)^{2} - 3^{2} + 11 = (x-3)^{2} + 2,

So sehen wir dass der Graph von der Funktion \displaystyle y = (x-3)^{2} + 2 der Graph von der Funktion \displaystyle y=x^{2} ist, nur zwei Einheiten nach oben, und drei Einheiten nach rechts verschoben (Sehen Sie 8a und 8b).


 
The graph of f(x) = x² The graph of f(x) = x² - 6x + 11
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_2.3:8c“