Lösung 4.4:2f
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 2: | Zeile 2: | ||
has two solutions for <math>0\le 3x\le 2\pi\,</math>, | has two solutions for <math>0\le 3x\le 2\pi\,</math>, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>3x = \frac{\pi}{2} + \frac{\pi}{4} = \frac{3\pi}{4}\qquad\text{and}\qquad 3x = \pi + \frac{\pi}{4} = \frac{5\pi}{4}\,\textrm{.}</math>}} |
[[Image:4_4_2_f.gif|center]] | [[Image:4_4_2_f.gif|center]] | ||
| Zeile 8: | Zeile 8: | ||
We obtain the other solutions by adding multiples of <math>2\pi</math>, | We obtain the other solutions by adding multiples of <math>2\pi</math>, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>3x = \frac{3\pi}{4} + 2n\pi\qquad\text{and}\qquad 3x = \frac{5\pi}{4} + 2n\pi\,,</math>}} |
i.e. | i.e. | ||
| - | {{ | + | {{Abgesetzte Formel||<math>x = \frac{\pi}{4} + \frac{2}{3}n\pi\qquad\text{and}\qquad x = \frac{5\pi}{12} + \frac{2}{3}n\pi\,,</math>}} |
where ''n'' is an arbitrary integer. | where ''n'' is an arbitrary integer. | ||
Version vom 08:58, 22. Okt. 2008
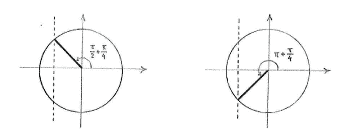
Using the unit circle shows that the equation \displaystyle \cos 3x = -1/\!\sqrt{2} has two solutions for \displaystyle 0\le 3x\le 2\pi\,,
| \displaystyle 3x = \frac{\pi}{2} + \frac{\pi}{4} = \frac{3\pi}{4}\qquad\text{and}\qquad 3x = \pi + \frac{\pi}{4} = \frac{5\pi}{4}\,\textrm{.} |
We obtain the other solutions by adding multiples of \displaystyle 2\pi,
| \displaystyle 3x = \frac{3\pi}{4} + 2n\pi\qquad\text{and}\qquad 3x = \frac{5\pi}{4} + 2n\pi\,, |
i.e.
| \displaystyle x = \frac{\pi}{4} + \frac{2}{3}n\pi\qquad\text{and}\qquad x = \frac{5\pi}{12} + \frac{2}{3}n\pi\,, |
where n is an arbitrary integer.