Lösung 4.4:4
Aus Online Mathematik Brückenkurs 1
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | The idea is first to find the general solution to the equation and then to see which angles lie between | + | The idea is first to find the general solution to the equation and then to see which angles lie between <math>0^{\circ}</math> and <math>360^{\circ}\,</math>. |
| - | <math>0^{\circ }</math> | + | |
| - | and | + | |
| - | <math>360^{\circ }</math>. | + | |
| - | If we start by considering the expression | + | If we start by considering the expression <math>2v+10^{\circ}</math> as an unknown, then we have a usual basic trigonometric equation. One solution which we can see directly is |
| - | <math> | + | |
| - | as an unknown, then we have a usual basic trigonometric equation. One solution which we can see directly is | + | |
| + | {{Displayed math||<math>2v + 10^{\circ} = 110^{\circ}\,\textrm{.}</math>}} | ||
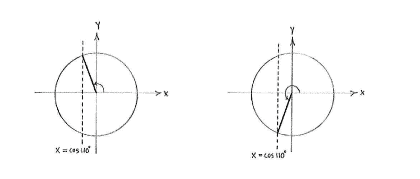
| - | + | There is then a further solution which satisfies <math>0^{\circ}\le 2v + 10^{\circ}\le 360^{\circ}</math>, where <math>2v+10^{\circ}</math> lies in the third quadrant and makes the same angle with the negative ''y''-axis as <math>100^{\circ}</math> makes with the positive ''y''-axis, i.e. <math>2v + 10^{\circ}</math> makes an angle <math>110^{\circ} - 90^{\circ} = 20^{\circ}</math> | |
| - | + | with the negative ''y''-axis and consequently | |
| - | + | ||
| - | There is then a further solution which satisfies | + | |
| - | <math>0^{\circ }\le | + | |
| - | <math> | + | |
| - | lies in the third quadrant and makes the same angle with the negative y-axis as | + | |
| - | <math> | + | |
| - | makes with the positive | + | |
| - | + | ||
| - | -axis, i.e. | + | |
| - | <math> | + | |
| - | makes an angle | + | |
| - | <math> | + | |
| - | with the negative | + | |
| - | + | ||
| - | -axis and consequently | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>2v + 10^{\circ} = 270^{\circ} - 20^{\circ} = 250^{\circ}\,\textrm{.}</math>}} | ||
[[Image:4_4_4.gif|center]] | [[Image:4_4_4.gif|center]] | ||
| - | |||
| - | |||
| - | There is then a further solution which satisfies | ||
| - | <math>0^{\circ }\le \text{2}v+\text{1}0^{\circ }\le \text{36}0^{\circ }</math>, where | ||
| - | <math>\text{2}v+\text{1}0^{\circ }\text{ }</math> | ||
| - | lies in the third quadrant and makes the same angle with the negative y-axis as | ||
| - | <math>\text{1}00^{\circ }</math> | ||
| - | makes with the positive | ||
| - | <math>y</math> | ||
| - | -axis, i.e. | ||
| - | <math>\text{2}v+\text{1}0^{\circ }\text{ }</math> | ||
| - | makes an angle | ||
| - | <math>\text{11}0^{\circ }-\text{9}0^{\circ }=\text{2}0^{\circ }~\text{ }</math> | ||
| - | with the negative | ||
| - | <math>y</math> | ||
| - | -axis and consequently | ||
| - | |||
| - | |||
| - | <math>\text{2}v+\text{1}0^{\circ }=270^{\circ }-20^{\circ }=250^{\circ }</math> | ||
| - | |||
| - | |||
| - | FIGURE1 FIGURE2 | ||
Now it is easy to write down the general solution, | Now it is easy to write down the general solution, | ||
| + | {{Displayed math||<math>\left\{\begin{align} 2v + 10^{\circ} &= 110^{\circ} + n\cdot 360^{\circ}\quad\text{and}\\[5pt] 2v + 10^{\circ} &= 250^{\circ} + n\cdot 360^{\circ}\,,\end{align}\right.</math>}} | ||
| - | + | and if we make ''v'' the subject, we get | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | and if we make | + | |
| - | + | ||
| - | the subject, we get | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>\left\{\begin{align} v &= 50^{\circ} + n\cdot 180^{\circ}\quad\text{and}\\[5pt] v &= 120^{\circ} + n\cdot 180^{\circ}\end{align}\right.</math>}} | ||
| - | + | For different values of the integers ''n'', we see that the corresponding solutions are: | |
| - | + | ||
| - | n | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {| align="center" | ||
| + | |align="center"|<math>\cdots\cdots</math> | ||
| + | || | ||
| + | |align="center"|<math>\cdots\cdots</math> | ||
| + | || | ||
| + | |align="center"|<math>\cdots\cdots</math> | ||
| + | |- | ||
| + | |align="left"|<math>n=-2:</math> | ||
| + | |width="20px"| | ||
| + | |align="left"|<math>v = 50^{\circ} - 2\cdot 180^{\circ} = -310^{\circ}</math> | ||
| + | |width="20px"| | ||
| + | |align="left"|<math>v = 120^{\circ } - 2\cdot 180^{\circ} = -240^{\circ}</math> | ||
| + | |- | ||
| + | |align="left"|<math>n=-1:</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 50^{\circ} - 1\cdot 180^{\circ} = -130^{\circ}</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 120^{\circ} - 1\cdot 180^{\circ} = -60^{\circ}</math> | ||
| + | |- | ||
| + | |align="left"|<math>n=0:</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 50^{\circ} + 0\cdot 180^{\circ} = 50^{\circ}</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 120^{\circ} + 0\cdot 180^{\circ} = 120^{\circ}</math> | ||
| + | |- | ||
| + | |align="left"|<math>n=1:</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 50^{\circ} + 1\cdot 180^{\circ} = 230^{\circ}</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 120^{\circ} + 1\cdot 180^{\circ} = 300^{\circ}</math> | ||
| + | |- | ||
| + | |align="left"|<math>n=2:</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 50^{\circ} + 2\cdot 180^{\circ} = 410^{\circ}</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 120^{\circ} + 2\cdot 180^{\circ} = 480^{\circ}</math> | ||
| + | |- | ||
| + | |align="left"|<math>n=3:</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 50^{\circ} + 3\cdot 180^{\circ} = 590^{\circ}</math> | ||
| + | || | ||
| + | |align="left"|<math>v = 120^{\circ} + 3\cdot 180^{\circ} = 660^{\circ}</math> | ||
| + | |- | ||
| + | |align="center"|<math>\cdots\cdots</math> | ||
| + | || | ||
| + | |align="center"|<math>\cdots\cdots</math> | ||
| + | || | ||
| + | |align="center"|<math>\cdots\cdots</math> | ||
| + | |} | ||
| - | From the table, we see that the solutions that are between | ||
| - | <math>0^{\circ }</math> | ||
| - | and | ||
| - | <math>360^{\circ }</math> | ||
| - | are | ||
| + | From the table, we see that the solutions that are between <math>0^{\circ}</math> and <math>360^{\circ}</math> are | ||
| - | <math>v=50,\quad v=120^{\circ },\quad v=230^{\circ } | + | {{Displayed math||<math>v = 50^{\circ},\quad v=120^{\circ },\quad v=230^{\circ}\quad\text{and}\quad v=300^{\circ}\,\textrm{.}</math>}} |
| - | and | + | |
| - | + | ||
Version vom 13:42, 13. Okt. 2008
The idea is first to find the general solution to the equation and then to see which angles lie between \displaystyle 0^{\circ} and \displaystyle 360^{\circ}\,.
If we start by considering the expression \displaystyle 2v+10^{\circ} as an unknown, then we have a usual basic trigonometric equation. One solution which we can see directly is
There is then a further solution which satisfies \displaystyle 0^{\circ}\le 2v + 10^{\circ}\le 360^{\circ}, where \displaystyle 2v+10^{\circ} lies in the third quadrant and makes the same angle with the negative y-axis as \displaystyle 100^{\circ} makes with the positive y-axis, i.e. \displaystyle 2v + 10^{\circ} makes an angle \displaystyle 110^{\circ} - 90^{\circ} = 20^{\circ} with the negative y-axis and consequently
Now it is easy to write down the general solution,
and if we make v the subject, we get
For different values of the integers n, we see that the corresponding solutions are:
| \displaystyle \cdots\cdots | \displaystyle \cdots\cdots | \displaystyle \cdots\cdots | ||
| \displaystyle n=-2: | \displaystyle v = 50^{\circ} - 2\cdot 180^{\circ} = -310^{\circ} | \displaystyle v = 120^{\circ } - 2\cdot 180^{\circ} = -240^{\circ} | ||
| \displaystyle n=-1: | \displaystyle v = 50^{\circ} - 1\cdot 180^{\circ} = -130^{\circ} | \displaystyle v = 120^{\circ} - 1\cdot 180^{\circ} = -60^{\circ} | ||
| \displaystyle n=0: | \displaystyle v = 50^{\circ} + 0\cdot 180^{\circ} = 50^{\circ} | \displaystyle v = 120^{\circ} + 0\cdot 180^{\circ} = 120^{\circ} | ||
| \displaystyle n=1: | \displaystyle v = 50^{\circ} + 1\cdot 180^{\circ} = 230^{\circ} | \displaystyle v = 120^{\circ} + 1\cdot 180^{\circ} = 300^{\circ} | ||
| \displaystyle n=2: | \displaystyle v = 50^{\circ} + 2\cdot 180^{\circ} = 410^{\circ} | \displaystyle v = 120^{\circ} + 2\cdot 180^{\circ} = 480^{\circ} | ||
| \displaystyle n=3: | \displaystyle v = 50^{\circ} + 3\cdot 180^{\circ} = 590^{\circ} | \displaystyle v = 120^{\circ} + 3\cdot 180^{\circ} = 660^{\circ} | ||
| \displaystyle \cdots\cdots | \displaystyle \cdots\cdots | \displaystyle \cdots\cdots |
From the table, we see that the solutions that are between \displaystyle 0^{\circ} and \displaystyle 360^{\circ} are