Lösung 4.3:3e

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
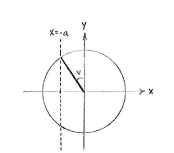
The angle
-
<center> [[Image:4_3_3e.gif]] </center>
+
<math>\frac{\pi }{2}+v</math>
-
{{NAVCONTENT_STOP}}
+
makes the same angle with the positive
 +
<math>y</math>
 +
-axis as the angle
 +
<math>v</math>
 +
makes with the positive
 +
<math>x</math>
 +
-axis, and hence we see that the
 +
<math>x</math>
 +
-coordinate for
 +
<math>\frac{\pi }{2}+v</math>
 +
is equal to the
 +
<math>y</math>
 +
-coordinate for
 +
<math>v</math>, but with a change of sign, i.e.
 +
 
 +
 
 +
<math>\cos \left( \frac{\pi }{2}+v \right)=-\sin v=-a</math>
[[Image:4_3_3_e-1.gif|center]][[Image:4_3_3_e-2.gif|center]]
[[Image:4_3_3_e-1.gif|center]][[Image:4_3_3_e-2.gif|center]]
 +
 +
 +
angle
 +
<math>v</math>
 +
angle
 +
<math>\frac{\pi }{2}+v</math>

Version vom 09:12, 10. Okt. 2008

The angle \displaystyle \frac{\pi }{2}+v makes the same angle with the positive \displaystyle y -axis as the angle \displaystyle v makes with the positive \displaystyle x -axis, and hence we see that the \displaystyle x -coordinate for \displaystyle \frac{\pi }{2}+v is equal to the \displaystyle y -coordinate for \displaystyle v, but with a change of sign, i.e.


\displaystyle \cos \left( \frac{\pi }{2}+v \right)=-\sin v=-a


angle \displaystyle v angle \displaystyle \frac{\pi }{2}+v

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.3:3e“