Lösung 4.2:3d
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | In order to get an angle between | + | In order to get an angle between <math>0</math> and <math>\text{2}\pi</math>, we subtract <math>2\pi</math> from <math>{7\pi }/{2}\,</math>, which also leaves the cosine value unchanged |
| - | <math>0</math> | + | |
| - | and | + | |
| - | <math>\text{2}\pi </math>, we subtract | + | |
| - | <math> | + | |
| - | from | + | |
| - | <math>{7\pi }/{2}\ | + | |
| - | , which also leaves the cosine value unchanged | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>\cos\frac{7\pi}{2} = \cos\Bigl(\frac{7\pi}{2}-2\pi\Bigr) = \cos\frac{3\pi}{2}\,\textrm{.}</math>}} | ||
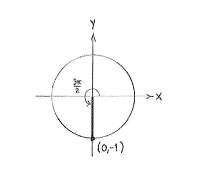
| + | When we draw a line which makes an angle <math>3\pi/2</math> with the positive ''x''-axis, we get the negative ''y''-axis and we see that this line cuts the unit circle at the point (0,-1). The ''x''-coordinate of the intersection point is thus | ||
| + | <math>0</math> and hence <math>\cos (7\pi/2) = \cos (3\pi/2) = 0\,</math>. | ||
[[Image:4_2_3_d.gif|center]] | [[Image:4_2_3_d.gif|center]] | ||
Version vom 07:57, 9. Okt. 2008
In order to get an angle between \displaystyle 0 and \displaystyle \text{2}\pi, we subtract \displaystyle 2\pi from \displaystyle {7\pi }/{2}\,, which also leaves the cosine value unchanged
When we draw a line which makes an angle \displaystyle 3\pi/2 with the positive x-axis, we get the negative y-axis and we see that this line cuts the unit circle at the point (0,-1). The x-coordinate of the intersection point is thus \displaystyle 0 and hence \displaystyle \cos (7\pi/2) = \cos (3\pi/2) = 0\,.