Lösung 2.3:8b

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K
Zeile 4: Zeile 4:
{| align="center"
{| align="center"
-
||[[Image:2_3_8_b-1.gif|center]]
+
|align="center"|[[Image:2_3_8_b-1.gif|center]]
|width="10px"| 
|width="10px"| 
-
||[[Image:2_3_8_b-2.gif|center]]
+
|align="center"|[[Image:2_3_8_b-2.gif|center]]
|-
|-
-
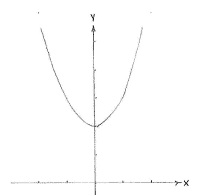
||<small>The graph of ''f''(''x'')&nbsp;=&nbsp;''x''²&nbsp;+&nbsp;2</small>
+
|align="center"|<small>The graph of ''f''(''x'')&nbsp;=&nbsp;''x''²&nbsp;+&nbsp;2</small>
||
||
-
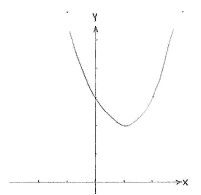
||<small>The graph of ''f''(''x'')&nbsp;=&nbsp;(''x''&nbsp;-&nbsp;1)²&nbsp;+&nbsp;2</small>
+
|align="center"|<small>The graph of ''f''(''x'')&nbsp;=&nbsp;(''x''&nbsp;-&nbsp;1)²&nbsp;+&nbsp;2</small>
|}
|}

Version vom 12:57, 29. Sep. 2008

As a starting point, we can take the curve \displaystyle y=x^{2}+2 which is a parabola with a minimum at (0,2) and is sketched further down. Compared with that curve, \displaystyle y = (x-1)^{2}+2 is the same curve in which we must consistently choose x to be one unit greater in order to get the same y-value. The curve \displaystyle y = (x-1)^{2}+2 is thus shifted one unit to the right compared with \displaystyle y=x^{2}+2.


 
The graph of f(x) = x² + 2 The graph of f(x) = (x - 1)² + 2
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_2.3:8b“