Lösung 4.2:4e
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (Lösning 4.2:4e moved to Solution 4.2:4e: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
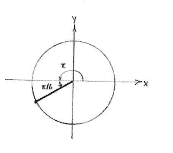
| - | {{ | + | If we write the angle |
| - | < | + | <math>\frac{7\pi }{6}</math> |
| - | {{ | + | as |
| + | |||
| + | <math>\frac{7\pi }{6}=\frac{6\pi +\pi }{6}=\pi +\frac{\pi }{6}</math> | ||
| + | |||
| + | we see that the angle | ||
| + | <math>\frac{7\pi }{6}</math> | ||
| + | on a unit circle is in the third quadrant and makes an angle | ||
| + | <math>\frac{\pi }{6}</math> | ||
| + | with the negative | ||
| + | <math>x</math> | ||
| + | -axis. | ||
| + | |||
[[Image:4_2_4_e1.gif|center]] | [[Image:4_2_4_e1.gif|center]] | ||
| + | |||
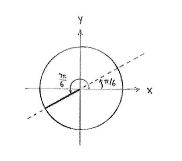
| + | Geometrically, | ||
| + | <math>\tan \frac{7\pi }{6}</math> | ||
| + | is defined as the gradient of the line having an angle | ||
| + | <math>\frac{7\pi }{6}</math> | ||
| + | and, because this line has the same slope as the line having angle | ||
| + | <math>\frac{\pi }{6}</math>, we have that | ||
| + | |||
| + | <math>\tan \frac{7\pi }{6}=\tan \frac{\pi }{6}=\frac{\sin \frac{\pi }{6}}{\cos \frac{\pi }{6}}=\frac{\frac{1}{2}}{\frac{\sqrt{3}}{2}}=\frac{1}{\sqrt{3}}</math> | ||
| + | |||
| + | |||
[[Image:4_2_4_e2.gif|center]] | [[Image:4_2_4_e2.gif|center]] | ||
Version vom 13:23, 28. Sep. 2008
If we write the angle \displaystyle \frac{7\pi }{6} as
\displaystyle \frac{7\pi }{6}=\frac{6\pi +\pi }{6}=\pi +\frac{\pi }{6}
we see that the angle \displaystyle \frac{7\pi }{6} on a unit circle is in the third quadrant and makes an angle \displaystyle \frac{\pi }{6} with the negative \displaystyle x -axis.
Geometrically, \displaystyle \tan \frac{7\pi }{6} is defined as the gradient of the line having an angle \displaystyle \frac{7\pi }{6} and, because this line has the same slope as the line having angle \displaystyle \frac{\pi }{6}, we have that
\displaystyle \tan \frac{7\pi }{6}=\tan \frac{\pi }{6}=\frac{\sin \frac{\pi }{6}}{\cos \frac{\pi }{6}}=\frac{\frac{1}{2}}{\frac{\sqrt{3}}{2}}=\frac{1}{\sqrt{3}}