Lösung 4.2:4b
Aus Online Mathematik Brückenkurs 1
K (Lösning 4.2:4b moved to Solution 4.2:4b: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | {{ | + | We start by subtracting |
| - | < | + | <math>2\pi </math> |
| - | {{ | + | from |
| - | {{ | + | <math>\frac{11\pi }{3}</math>, so that we get an angle between |
| - | < | + | <math>o</math> |
| - | {{ | + | and |
| + | <math>2\pi </math>. This doesn't change the cosine value | ||
| + | |||
| + | |||
| + | <math>\cos \frac{11\pi }{3}=\cos \left( \frac{11\pi }{3}-2\pi \right)=\cos \frac{5\pi }{3}</math> | ||
| + | |||
| + | |||
| + | Then, by rewriting | ||
| + | <math>\frac{5\pi }{3}</math> | ||
| + | as a sum of | ||
| + | <math>\pi </math> | ||
| + | - and | ||
| + | <math>\frac{\pi }{2}</math> | ||
| + | -terms | ||
| + | |||
| + | |||
| + | <math>\frac{5\pi }{3}=\frac{3\pi +\frac{3}{2}\pi +\frac{1}{2}\pi }{3}=\pi +\frac{\pi }{2}+\frac{\pi }{6}</math> | ||
| + | |||
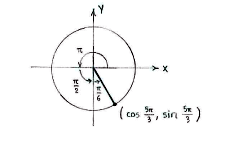
| + | we see that | ||
| + | <math>\frac{5\pi }{3}</math> | ||
| + | is an angle in the fourth quadrant which makes an angle | ||
| + | <math>\frac{\pi }{6}</math> | ||
| + | with the negative | ||
| + | <math>y</math> | ||
| + | -axis. | ||
| + | |||
| + | |||
[[Image:4_2_4b1.gif]] | [[Image:4_2_4b1.gif]] | ||
| + | |||
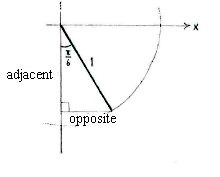
| + | With the help of a triangle and a little trigonometry, we can determine the coordinates for the point on a unit circle which corresponds to the angle | ||
| + | <math>\frac{5\pi }{3}</math> . | ||
| + | |||
| + | |||
[[Image:4_2_4_b2.gif]] | [[Image:4_2_4_b2.gif]] | ||
| + | |||
| + | The point has coordinates | ||
| + | <math>\left( \frac{1}{2} \right.,\left. -\frac{\sqrt{3}}{2} \right)</math> | ||
| + | and | ||
| + | <math>\cos \frac{11\pi }{3}=\cos \frac{5\pi }{3}=\frac{1}{2}</math>. | ||
Version vom 13:05, 28. Sep. 2008
We start by subtracting \displaystyle 2\pi from \displaystyle \frac{11\pi }{3}, so that we get an angle between \displaystyle o and \displaystyle 2\pi . This doesn't change the cosine value
\displaystyle \cos \frac{11\pi }{3}=\cos \left( \frac{11\pi }{3}-2\pi \right)=\cos \frac{5\pi }{3}
Then, by rewriting
\displaystyle \frac{5\pi }{3}
as a sum of
\displaystyle \pi
- and
\displaystyle \frac{\pi }{2}
-terms
\displaystyle \frac{5\pi }{3}=\frac{3\pi +\frac{3}{2}\pi +\frac{1}{2}\pi }{3}=\pi +\frac{\pi }{2}+\frac{\pi }{6}
we see that \displaystyle \frac{5\pi }{3} is an angle in the fourth quadrant which makes an angle \displaystyle \frac{\pi }{6} with the negative \displaystyle y -axis.
With the help of a triangle and a little trigonometry, we can determine the coordinates for the point on a unit circle which corresponds to the angle \displaystyle \frac{5\pi }{3} .
The point has coordinates \displaystyle \left( \frac{1}{2} \right.,\left. -\frac{\sqrt{3}}{2} \right) and \displaystyle \cos \frac{11\pi }{3}=\cos \frac{5\pi }{3}=\frac{1}{2}.