Lösung 4.2:3a

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 4.2:3a moved to Solution 4.2:3a: Robot: moved page)
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
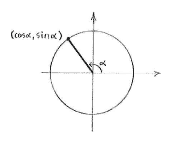
A useful technique for calculating the value of a trigonometric function for angles that don't lie between
-
<center> [[Image:4_2_3a.gif]] </center>
+
<math>0</math>
-
{{NAVCONTENT_STOP}}
+
and
 +
<math>{\pi }/{2}\;</math>
 +
is to use the unit circle. If we draw a line which starts at the origin and makes a certain angle relative to the positive part of the
 +
<math>x</math>
 +
-axis, we can see that the cosine of that angle is the
 +
<math>x</math>
 +
-coordinate of the point of intersection between the line and the unit circle. In the same way, the sine of the angle is the
 +
<math>y</math>
 +
-coordinate of the intersection point.
 +
 
 +
 
[[Image:4_2_3_a1.gif|center]]
[[Image:4_2_3_a1.gif|center]]
 +
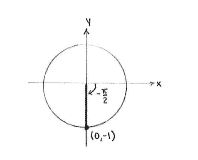
In this case, we see immediately that
 +
<math>\text{sin}\left( -\frac{\pi }{2} \right)\text{ }=\text{ }-\text{1}</math>.
 +
 +
 +
[[Image:4_2_3_a2.gif|center]]
[[Image:4_2_3_a2.gif|center]]

Version vom 11:47, 28. Sep. 2008

A useful technique for calculating the value of a trigonometric function for angles that don't lie between \displaystyle 0 and \displaystyle {\pi }/{2}\; is to use the unit circle. If we draw a line which starts at the origin and makes a certain angle relative to the positive part of the \displaystyle x -axis, we can see that the cosine of that angle is the \displaystyle x -coordinate of the point of intersection between the line and the unit circle. In the same way, the sine of the angle is the \displaystyle y -coordinate of the intersection point.


In this case, we see immediately that \displaystyle \text{sin}\left( -\frac{\pi }{2} \right)\text{ }=\text{ }-\text{1}.


Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.2:3a“