Lösung 2.2:8b

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 2.2:8b moved to Solution 2.2:8b: Robot: moved page)
Zeile 1: Zeile 1:
 +
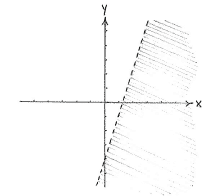
A point whose coordinates satisfy
 +
<math>y<3x-4</math>
 +
has a
 +
<math>y</math>
 +
-coordinate which is less than that of a point lying on the line
 +
<math>y=3x-4</math>
 +
and having the same
 +
<math>x</math>
 +
-coordinate. This means that the area we should shade consists of all points below the line
 +
<math>y=3x-4</math>.
 +
{{NAVCONTENT_START}}
{{NAVCONTENT_START}}
-
<center> [[Image:2_2_8b.gif]] </center>
+
 
{{NAVCONTENT_STOP}}
{{NAVCONTENT_STOP}}
[[Image:2_2_8_b.gif|center]]
[[Image:2_2_8_b.gif|center]]
 +
 +
We can draw the line
 +
<math>y=3x-4</math>
 +
by choosing two x-values, for example
 +
<math>x=0</math>
 +
and
 +
<math>x=1</math>, using the equation of the line to calculate the corresponding y-coordinates,
 +
<math>y=3\centerdot 0-4=-4</math>
 +
and
 +
<math>y=3\centerdot 1-4=-1</math>
 +
respectively, and then draw a straight line between the two points that we have obtained.

Version vom 12:34, 18. Sep. 2008

A point whose coordinates satisfy \displaystyle y<3x-4 has a \displaystyle y -coordinate which is less than that of a point lying on the line \displaystyle y=3x-4 and having the same \displaystyle x -coordinate. This means that the area we should shade consists of all points below the line \displaystyle y=3x-4.

We can draw the line \displaystyle y=3x-4 by choosing two x-values, for example \displaystyle x=0 and \displaystyle x=1, using the equation of the line to calculate the corresponding y-coordinates, \displaystyle y=3\centerdot 0-4=-4 and \displaystyle y=3\centerdot 1-4=-1 respectively, and then draw a straight line between the two points that we have obtained.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_2.2:8b“