Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 4.2:1a

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
Zeile 1: Zeile 1:
-
Die Definition von Tangens ist
+
Die Definition des Tangens ist
{| width="100%"
{| width="100%"
Zeile 10: Zeile 10:
{{Abgesetzte Formel||<math>\tan 27^{\circ} = \frac{x}{13}</math>}}
{{Abgesetzte Formel||<math>\tan 27^{\circ} = \frac{x}{13}</math>}}
-
und also ist <math>x = 13\cdot \tan 27^{\circ}\,</math>.
+
also ist <math>x = 13\cdot \tan 27^{\circ}\,</math>.
Hinweis: mit einem Taschenrechner können wir ''x'' bestimmen;
Hinweis: mit einem Taschenrechner können wir ''x'' bestimmen;
{{Abgesetzte Formel||<math>x = 13\cdot\tan 27^{\circ} \approx 6\textrm{.}62\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>x = 13\cdot\tan 27^{\circ} \approx 6\textrm{.}62\,\textrm{.}</math>}}

Version vom 17:12, 23. Jul. 2009

Die Definition des Tangens ist

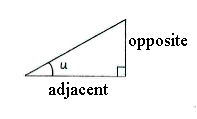
tanu=AnkatheteGegenkathete Image:4_2_1_a.gif

In unseren Fall haben wir

tan27=x13

also ist x=13tan27.

Hinweis: mit einem Taschenrechner können wir x bestimmen;

x=13tan276.62.
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.2:1a“