Lösung 4.3:2a

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (hat „Solution 4.3:2a“ nach „Lösung 4.3:2a“ verschoben: Robot: moved page)
Zeile 1: Zeile 1:
-
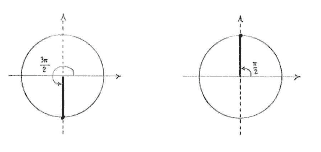
On the unit circle, the angle <math>3\pi/2</math> corresponds to the point (0,-1), and the angle in the interval from <math>0</math> to <math>\pi</math> which has the same cosine value as <math>3\pi/2</math>, i.e. the ''x''-coordinate <math>0</math>, is the angle <math>v = \pi/2\,</math>.
+
Am Einheitskreis entspricht der Winkel <math>3\pi/2</math> den Punkt (0,-1), und der Punkt zwischen <math>0</math> und <math>\pi</math> der denselben Kosinus wie <math>3\pi/2</math> hat, ist <math>v = \pi/2\,</math>, nachdem dieser Winkel am Einheitskreis auch die ''x''-Koordinate 0 hat.
[[Image:4_3_2_a.gif||center]]
[[Image:4_3_2_a.gif||center]]

Version vom 11:32, 5. Apr. 2009

Am Einheitskreis entspricht der Winkel \displaystyle 3\pi/2 den Punkt (0,-1), und der Punkt zwischen \displaystyle 0 und \displaystyle \pi der denselben Kosinus wie \displaystyle 3\pi/2 hat, ist \displaystyle v = \pi/2\,, nachdem dieser Winkel am Einheitskreis auch die x-Koordinate 0 hat.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.3:2a“