Lösung 4.2:4b

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (hat „Solution 4.2:4b“ nach „Lösung 4.2:4b“ verschoben: Robot: moved page)
Zeile 1: Zeile 1:
-
We start by subtracting <math>2\pi</math> from <math>11\pi/3</math>, so that we get an angle between <math>0</math> and <math>2\pi </math>. This doesn't change the cosine value
+
Zuerst subtrahieren wir <math>2\pi</math> von <math>11\pi/3</math>, sodass wir einen Winkel zwischen <math>0</math> und <math>2\pi </math> erhalten. Dies ändert nicht den Wert des Kosinus.
{{Abgesetzte Formel||<math>\cos\frac{11\pi}{3} = \cos\Bigl(\frac{11\pi}{3}-2\pi\Bigr) = \cos\frac{5\pi}{3}\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>\cos\frac{11\pi}{3} = \cos\Bigl(\frac{11\pi}{3}-2\pi\Bigr) = \cos\frac{5\pi}{3}\,\textrm{.}</math>}}
-
Then, by rewriting <math>5\pi/3</math> as a sum of <math>\pi</math>- and <math>\pi/2</math>-terms,
+
Danach schreiben wir <math>5\pi/3</math> wie eine Summe von <math>\pi</math>- und <math>\pi/2</math>-Termen,
{{Abgesetzte Formel||<math>\frac{5\pi}{3} = \frac{3\pi +\dfrac{3}{2}\pi +\dfrac{1}{2}\pi}{3} = \pi + \frac{\pi}{2} + \frac{\pi}{6}</math>}}
{{Abgesetzte Formel||<math>\frac{5\pi}{3} = \frac{3\pi +\dfrac{3}{2}\pi +\dfrac{1}{2}\pi}{3} = \pi + \frac{\pi}{2} + \frac{\pi}{6}</math>}}
-
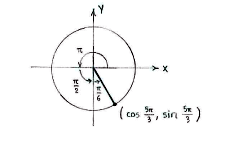
we see that <math>5\pi/3</math> is an angle in the fourth quadrant which makes an angle <math>\pi/6</math> with the negative ''y''-axis.
+
So sehen wir dass <math>5\pi/3</math> im vierten Quadrant liegt, und den Winkel <math>\pi/6</math> mit der negativen ''y''-Achse bildet.
[[Image:4_2_4b1.gif||center]]
[[Image:4_2_4b1.gif||center]]
-
With the help of an auxiliary triangle and a little trigonometry, we can determine the coordinates for the point on a unit circle which corresponds to the angle
+
Mit ein Wenig Trigonometrie berechnen wir den Punkt am Einheitskreis, der den Winkel <math>5\pi/3\,</math> entspricht,
-
<math>5\pi/3\,</math>.
+
{| width="100%"
{| width="100%"
|width="50%" align="center"|[[Image:4_2_4_b2.gif]]
|width="50%" align="center"|[[Image:4_2_4_b2.gif]]
-
|width="50%" align="left"|<math>\begin{align}\text{opposite} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{adjacent} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}</math>
+
|width="50%" align="left"|<math>\begin{align}\text{Gegenkathete} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{Ankathete} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}</math>
|}
|}
-
The point has coordinates <math>(1/2,-\sqrt{3}/2)</math> and
+
Der Punkt hat also die Koordinaten <math>(1/2,-\sqrt{3}/2)</math> und
{{Abgesetzte Formel||<math>\cos \frac{11\pi}{3} = \cos\frac{5\pi}{3} = \frac{1}{2}\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>\cos \frac{11\pi}{3} = \cos\frac{5\pi}{3} = \frac{1}{2}\,\textrm{.}</math>}}

Version vom 15:03, 4. Apr. 2009

Zuerst subtrahieren wir \displaystyle 2\pi von \displaystyle 11\pi/3, sodass wir einen Winkel zwischen \displaystyle 0 und \displaystyle 2\pi erhalten. Dies ändert nicht den Wert des Kosinus.

\displaystyle \cos\frac{11\pi}{3} = \cos\Bigl(\frac{11\pi}{3}-2\pi\Bigr) = \cos\frac{5\pi}{3}\,\textrm{.}

Danach schreiben wir \displaystyle 5\pi/3 wie eine Summe von \displaystyle \pi- und \displaystyle \pi/2-Termen,

\displaystyle \frac{5\pi}{3} = \frac{3\pi +\dfrac{3}{2}\pi +\dfrac{1}{2}\pi}{3} = \pi + \frac{\pi}{2} + \frac{\pi}{6}

So sehen wir dass \displaystyle 5\pi/3 im vierten Quadrant liegt, und den Winkel \displaystyle \pi/6 mit der negativen y-Achse bildet.

Mit ein Wenig Trigonometrie berechnen wir den Punkt am Einheitskreis, der den Winkel \displaystyle 5\pi/3\, entspricht,

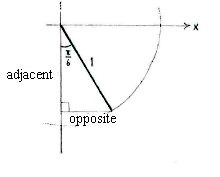
Image:4_2_4_b2.gif \displaystyle \begin{align}\text{Gegenkathete} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{Ankathete} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}

Der Punkt hat also die Koordinaten \displaystyle (1/2,-\sqrt{3}/2) und

\displaystyle \cos \frac{11\pi}{3} = \cos\frac{5\pi}{3} = \frac{1}{2}\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.2:4b“