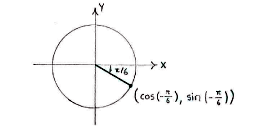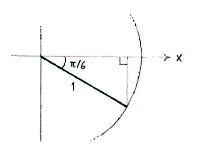Lösung 4.2:3f

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (hat „Solution 4.2:3f“ nach „Lösung 4.2:3f“ verschoben: Robot: moved page)
Zeile 1: Zeile 1:
-
The point on the unit circle which corresponds to the angle <math>-\pi/6</math> lies in the fourth quadrant.
+
Der Punkt auf den Einheitskreis der den Winkel <math>-\pi/6</math> entspricht liegt im vierten Quadrant.
[[Image:4_2_3_f1.gif||center]]
[[Image:4_2_3_f1.gif||center]]
-
As usual, <math>\cos (-\pi/6)</math> gives the ''x''-coordinate of the point of intersection between the angle's line and the unit circle. In order to determine this point, we introduce an auxiliary triangle in the fourth quadrant.
+
Wir betrachten ein Dreieck im vierten Quadrant
[[Image:4_2_3_f2.gif||center]]
[[Image:4_2_3_f2.gif||center]]
-
We can determine the edges in this triangle by simple trigonometry and then translate these over to the point's coordinates.
+
Wir berechnen zuerst die Katheten des Dreieckes, und danach die Koordinaten des Punktes.
{| width="100%"
{| width="100%"
|width="50%" align="center"|[[Image:4_2_3_f3.gif]]
|width="50%" align="center"|[[Image:4_2_3_f3.gif]]
-
|width="50%" align="left"|<math>\begin{align}\text{opposite} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{adjacent} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}</math>
+
|width="50%" align="left"|<math>\begin{align}\text{Gegenkathete} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{Ankathete} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}</math>
|}
|}
-
The coordinates of the point of intersection are <math>(\sqrt{3}/2,-1/2)</math> and in particular <math>\cos (-\pi/6) = \sqrt{3}/2\,</math>.
+
Die Koordinaten des Punktes auf den Einheitskreis entsprechend den Winkel <math>-\pi/6</math> sind also <math>(\sqrt{3}/2,-1/2)</math> und besonders ist <math>\cos (-\pi/6) = \sqrt{3}/2\,</math>.

Version vom 14:29, 4. Apr. 2009

Der Punkt auf den Einheitskreis der den Winkel \displaystyle -\pi/6 entspricht liegt im vierten Quadrant.

Wir betrachten ein Dreieck im vierten Quadrant

Wir berechnen zuerst die Katheten des Dreieckes, und danach die Koordinaten des Punktes.

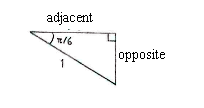
Image:4_2_3_f3.gif \displaystyle \begin{align}\text{Gegenkathete} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{Ankathete} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}

Die Koordinaten des Punktes auf den Einheitskreis entsprechend den Winkel \displaystyle -\pi/6 sind also \displaystyle (\sqrt{3}/2,-1/2) und besonders ist \displaystyle \cos (-\pi/6) = \sqrt{3}/2\,.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.2:3f“