Lösung 2.3:10c
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (hat „Solution 2.3:10c“ nach „Lösung 2.3:10c“ verschoben: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
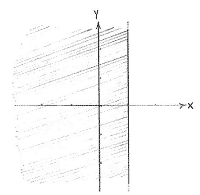
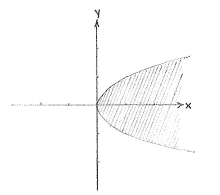
| - | + | Die Ungleichung <math>1\ge x\ge y^{2}</math> definiert das Gebiet das die beiden Ungleichungen <math>1\ge x</math> und <math>x\ge y^{2}</math> erfüllt. Die erste Ungleichung gibt dass unser Gebiet links von der Geaden <math>x=1</math> sein muss. Die andere Ungleichung ist genau wie die Ungleichung <math>y\ge x^{2}</math>, nur haben x und y Platz getauscht. Daher drehen wir die Parabel <math>y=x^{2}</math> sodass sie entlang der x-Achse wächst. | |
| Zeile 12: | Zeile 12: | ||
|} | |} | ||
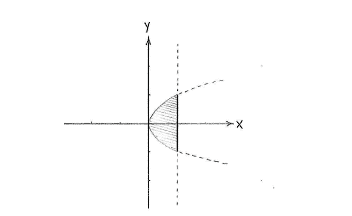
| - | + | Also bekommen wir das Gebiet zwischen der Parabel und der Gerade. | |
| - | + | ||
Version vom 21:13, 16. Mär. 2009
Die Ungleichung \displaystyle 1\ge x\ge y^{2} definiert das Gebiet das die beiden Ungleichungen \displaystyle 1\ge x und \displaystyle x\ge y^{2} erfüllt. Die erste Ungleichung gibt dass unser Gebiet links von der Geaden \displaystyle x=1 sein muss. Die andere Ungleichung ist genau wie die Ungleichung \displaystyle y\ge x^{2}, nur haben x und y Platz getauscht. Daher drehen wir die Parabel \displaystyle y=x^{2} sodass sie entlang der x-Achse wächst.
| The region 1 ≥ x | The region x ≥ y² |
Also bekommen wir das Gebiet zwischen der Parabel und der Gerade.
| The region 1 ≥ x ≥ y² |