Lösung 2.2:9b

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (hat „Solution 2.2:9b“ nach „Lösung 2.2:9b“ verschoben: Robot: moved page)
Zeile 1: Zeile 1:
-
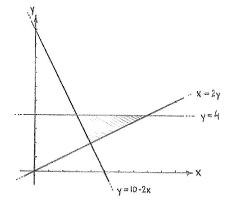
A first step is to draw the lines so that we get an overview of how the triangle looks like.
+
Zuerst zeichnen wir die Geraden, sodass wir sehen wie das Dreieck aussieht.
-
 
+
[[Image:2_2_9_b-1(3).gif|center]]
[[Image:2_2_9_b-1(3).gif|center]]
-
 
+
Die Ecken dieses Dreiecks, sind die Schnittpunkte des Geraden. Wir erhalten die Schnittstellen wenn wir die Gleichungen der Geraden für alle 3 Paare von geraden lösen.
-
The corner points of the triangle are the intersection points of the lines and we obtain those more exactly by choosing the equations of the lines in pairs and solving the resulting system of equations,
+
{{Abgesetzte Formel||<math>\left\{\begin{align} x&=2y,\\ y&=4,\end{align}\right.\qquad
{{Abgesetzte Formel||<math>\left\{\begin{align} x&=2y,\\ y&=4,\end{align}\right.\qquad
Zeile 12: Zeile 10:
\left\{\begin{align} y&=4,\\ y&=10-2y\,\textrm{.}\end{align}\right.</math>}}
\left\{\begin{align} y&=4,\\ y&=10-2y\,\textrm{.}\end{align}\right.</math>}}
-
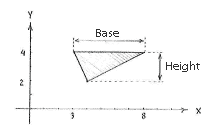
The first system of equations has the solution <math>(x,y) = (8,4)</math>, the second system has the solution <math>(x,y) = (4,2)</math>
+
Die Gleichungssysteme haben die Lösungen <math>(x,y) = (8,4)</math>, <math>(x,y) = (4,2)</math> und <math>(x,y) = (3,4)\,</math>.
-
and the third system <math>(x,y) = (3,4)\,</math>.
+
[[Image:2_2_9_b-2(3).gif|center]]
[[Image:2_2_9_b-2(3).gif|center]]
 +
Die Fläche des Dreiecks bekommen wir mit der Gleichung
-
If we go back to the area of the triangle, which is given by the formula
+
{{Abgesetzte Formel||<math>\text{Fläche} = \tfrac{1}{2}\cdot\text{(Basis)}\cdot\text{(Höhe),}</math>}}
-
 
+
-
{{Abgesetzte Formel||<math>\text{Area} = \tfrac{1}{2}\cdot\text{(base)}\cdot\text{(height),}</math>}}
+
-
we are completely free to choose which edge of the triangle we take as the base. Because one of the edges is parallel with the ''x''-axis, it seems best to choose that one as the base, since then we can easily read off the length of the base and the height as the difference in coordinates of the corner points.
+
Nachdem hier eine Kante mit der ''x''-Achse parallel ist, wählen wir diese Kante als Basis des Dreiecks.
Zeile 29: Zeile 25:
-
The base is given as the horizontal distance between (3,4) and (8,4),
+
Die Basis ist einfach der Unterschied zwischen den ''x''-Koordinaten (3,4) und (8,4),
{{Abgesetzte Formel||<math>\text{base} = 8-3 = 5</math>}}
{{Abgesetzte Formel||<math>\text{base} = 8-3 = 5</math>}}
-
and we obtain the height in the same way as the difference in the ''y''-direction between the base at <math>y=4</math> and the corner point (4,2),
+
Wir erhalten die Höhe des Dreiecks als der Unterschied zwischen den ''x''-Koordinaten von dem Punkt (4,2) und von der Gerade <math>y=4</math>
{{Abgesetzte Formel||<math>\text{height} = 4-2 = 2\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>\text{height} = 4-2 = 2\,\textrm{.}</math>}}
-
The area of the triangle is
+
Die Fläche des Dreiecks ist also
{{Abgesetzte Formel||<math>\text{Area} = \tfrac{1}{2}\cdot\text{(base)}\cdot\text{(height)} = \tfrac{1}{2}\cdot 5\cdot 2 = 5\,\text{u.a.}</math>}}
{{Abgesetzte Formel||<math>\text{Area} = \tfrac{1}{2}\cdot\text{(base)}\cdot\text{(height)} = \tfrac{1}{2}\cdot 5\cdot 2 = 5\,\text{u.a.}</math>}}

Version vom 13:30, 13. Mär. 2009

Zuerst zeichnen wir die Geraden, sodass wir sehen wie das Dreieck aussieht.

Die Ecken dieses Dreiecks, sind die Schnittpunkte des Geraden. Wir erhalten die Schnittstellen wenn wir die Gleichungen der Geraden für alle 3 Paare von geraden lösen.

\displaystyle \left\{\begin{align} x&=2y,\\ y&=4,\end{align}\right.\qquad

\left\{\begin{align} x&=2y,\\ y&=10-2y,\end{align}\right.\qquad \text{and}\qquad \left\{\begin{align} y&=4,\\ y&=10-2y\,\textrm{.}\end{align}\right.

Die Gleichungssysteme haben die Lösungen \displaystyle (x,y) = (8,4), \displaystyle (x,y) = (4,2) und \displaystyle (x,y) = (3,4)\,.


Die Fläche des Dreiecks bekommen wir mit der Gleichung

\displaystyle \text{Fläche} = \tfrac{1}{2}\cdot\text{(Basis)}\cdot\text{(Höhe),}

Nachdem hier eine Kante mit der x-Achse parallel ist, wählen wir diese Kante als Basis des Dreiecks.



Die Basis ist einfach der Unterschied zwischen den x-Koordinaten (3,4) und (8,4),

\displaystyle \text{base} = 8-3 = 5

Wir erhalten die Höhe des Dreiecks als der Unterschied zwischen den x-Koordinaten von dem Punkt (4,2) und von der Gerade \displaystyle y=4

\displaystyle \text{height} = 4-2 = 2\,\textrm{.}

Die Fläche des Dreiecks ist also

\displaystyle \text{Area} = \tfrac{1}{2}\cdot\text{(base)}\cdot\text{(height)} = \tfrac{1}{2}\cdot 5\cdot 2 = 5\,\text{u.a.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_2.2:9b“