Lösung 4.4:3c
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (hat „Solution 4.4:3c“ nach „Lösung 4.4:3c“ verschoben: Robot: moved page) |
Version vom 15:15, 22. Okt. 2008
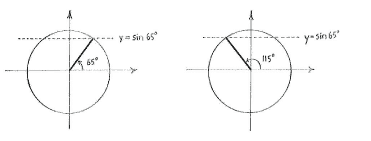
If we consider the entire expression \displaystyle x + 40^{\circ} as an unknown, we have a basic trigonometric equation and can, with the aid of the unit circle, see that there are two solutions to the equation for \displaystyle 0^{\circ}\le x+40^{\circ}\le 360^{\circ} namely \displaystyle x+40^{\circ} = 65^{\circ} and the symmetric solution \displaystyle x + 40^{\circ} = 180^{\circ} - 65^{\circ} = 115^{\circ}\,.
It is then easy to set up the general solution by adding multiples of \displaystyle 360^{\circ}\,,
| \displaystyle x + 40^{\circ} = 65^{\circ} + n\cdot 360^{\circ}\qquad\text{and}\qquad x + 40^{\circ} = 115^{\circ} + n\cdot 360^{\circ} |
for all integers n, which gives
| \displaystyle x = 25^{\circ} + n\cdot 360^{\circ}\qquad\text{and}\qquad x=75^{\circ} + n\cdot 360^{\circ}\,\textrm{.} |