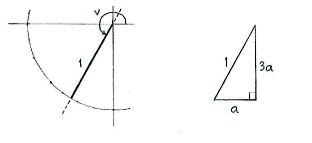Lösung 4.3:6c
Aus Online Mathematik Brückenkurs 1
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 10: | Zeile 10: | ||
If we now use the Pythagorean theorem on the triangle, we see that the horizontal side ''a'' satisfies | If we now use the Pythagorean theorem on the triangle, we see that the horizontal side ''a'' satisfies | ||
| - | {{ | + | {{Abgesetzte Formel||<math>a^2 + (3a)^2 = 1^2</math>}} |
which gives us that <math>10a^{2}=1</math> i.e. <math>a = 1/\!\sqrt{10}\,\textrm{.}</math> | which gives us that <math>10a^{2}=1</math> i.e. <math>a = 1/\!\sqrt{10}\,\textrm{.}</math> | ||
| Zeile 16: | Zeile 16: | ||
Thus, the angle ''v'''s ''x''-coordinate is <math>-1/\!\sqrt{10}</math> and ''y''-coordinate is <math>-3/\!\sqrt{10}</math>, i.e. | Thus, the angle ''v'''s ''x''-coordinate is <math>-1/\!\sqrt{10}</math> and ''y''-coordinate is <math>-3/\!\sqrt{10}</math>, i.e. | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
\cos v &= -\frac{1}{\sqrt{10}}\,,\\[5pt] | \cos v &= -\frac{1}{\sqrt{10}}\,,\\[5pt] | ||
\sin v &= -\frac{3}{\sqrt{10}}\,\textrm{.} | \sin v &= -\frac{3}{\sqrt{10}}\,\textrm{.} | ||
\end{align}</math>}} | \end{align}</math>}} | ||
Version vom 08:56, 22. Okt. 2008
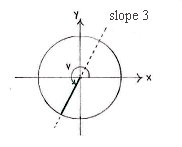
Because the angle \displaystyle v satisfies \displaystyle \pi \le v\le 3\pi/2\,, \displaystyle v belongs to the third quadrant in the unit circle. Furthermore, \displaystyle \tan v = 3 gives that the line which corresponds to the angle \displaystyle v has slope 3.
In the third quadrant, we can introduce a right-angled triangle in which the hypotenuse is 1 and the sides have a 3:1 ratio.
If we now use the Pythagorean theorem on the triangle, we see that the horizontal side a satisfies
| \displaystyle a^2 + (3a)^2 = 1^2 |
which gives us that \displaystyle 10a^{2}=1 i.e. \displaystyle a = 1/\!\sqrt{10}\,\textrm{.}
Thus, the angle v's x-coordinate is \displaystyle -1/\!\sqrt{10} and y-coordinate is \displaystyle -3/\!\sqrt{10}, i.e.
| \displaystyle \begin{align}
\cos v &= -\frac{1}{\sqrt{10}}\,,\\[5pt] \sin v &= -\frac{3}{\sqrt{10}}\,\textrm{.} \end{align} |