Lösung 4.3:2b

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel))
Zeile 1: Zeile 1:
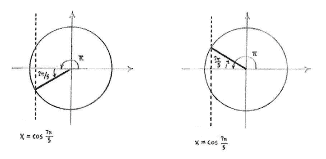
If we write the angle <math>\frac{7\pi }{5}</math> as
If we write the angle <math>\frac{7\pi }{5}</math> as
-
{{Displayed math||<math>\frac{7\pi}{5} = \frac{5\pi+2\pi}{5} = \pi + \frac{2\pi }{5}</math>}}
+
{{Abgesetzte Formel||<math>\frac{7\pi}{5} = \frac{5\pi+2\pi}{5} = \pi + \frac{2\pi }{5}</math>}}
we see that <math>7\pi/5</math> is an angle in the third quadrant.
we see that <math>7\pi/5</math> is an angle in the third quadrant.
Zeile 9: Zeile 9:
The angle between <math>0</math> and <math>\pi</math> which has the same ''x''-coordinate as the angle <math>7\pi/5</math>, and hence the same cosine value, is the reflection of the angle <math>7\pi/5</math> in the ''x''-axis, i.e.
The angle between <math>0</math> and <math>\pi</math> which has the same ''x''-coordinate as the angle <math>7\pi/5</math>, and hence the same cosine value, is the reflection of the angle <math>7\pi/5</math> in the ''x''-axis, i.e.
-
{{Displayed math||<math>v = \pi -\frac{2\pi}{5} = \frac{3\pi}{5}\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>v = \pi -\frac{2\pi}{5} = \frac{3\pi}{5}\,\textrm{.}</math>}}

Version vom 08:54, 22. Okt. 2008

If we write the angle \displaystyle \frac{7\pi }{5} as

\displaystyle \frac{7\pi}{5} = \frac{5\pi+2\pi}{5} = \pi + \frac{2\pi }{5}

we see that \displaystyle 7\pi/5 is an angle in the third quadrant.

The angle between \displaystyle 0 and \displaystyle \pi which has the same x-coordinate as the angle \displaystyle 7\pi/5, and hence the same cosine value, is the reflection of the angle \displaystyle 7\pi/5 in the x-axis, i.e.

\displaystyle v = \pi -\frac{2\pi}{5} = \frac{3\pi}{5}\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.3:2b“