Aus Online Mathematik Brückenkurs 1

Version vom 13:52, 8. Okt. 2008 (bearbeiten) Tek (Diskussion | Beiträge) K ← Zum vorherigen Versionsunterschied		Version vom 08:50, 22. Okt. 2008 (bearbeiten) (rückgängig) Tekbot (Diskussion | Beiträge) K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) Zum nächsten Versionsunterschied →
Zeile 8:		Zeile 8:
	In our case, this means that		In our case, this means that
-	{{Displayed math||<math>\tan 27^{\circ} = \frac{x}{13}</math>}}	+	{{Abgesetzte Formel||<math>\tan 27^{\circ} = \frac{x}{13}</math>}}
	which gives <math>x = 13\cdot \tan 27^{\circ}\,</math>.		which gives <math>x = 13\cdot \tan 27^{\circ}\,</math>.
Zeile 15:		Zeile 15:
	Note: Using a calculator, we can work out what ''x'' should be,		Note: Using a calculator, we can work out what ''x'' should be,
-	{{Displayed math||<math>x = 13\cdot\tan 27^{\circ} \approx 6\textrm{.}62\,\textrm{.}</math>}}	+	{{Abgesetzte Formel||<math>x = 13\cdot\tan 27^{\circ} \approx 6\textrm{.}62\,\textrm{.}</math>}}

---

Version vom 08:50, 22. Okt. 2008

The definition of the tangent states that

\displaystyle \tan u=\frac{\text{opposite}}{\text{adjacent}}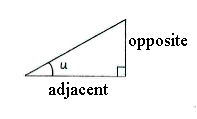

In our case, this means that

\displaystyle \tan 27^{\circ} = \frac{x}{13}

which gives \displaystyle x = 13\cdot \tan 27^{\circ}\,.

Note: Using a calculator, we can work out what x should be,

\displaystyle x = 13\cdot\tan 27^{\circ} \approx 6\textrm{.}62\,\textrm{.}