Lösung 4.1:7a

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel))
Zeile 1: Zeile 1:
As the equation stands, it is difficult directly to know anything about the circle, but if we complete the square and combine ''x''- and ''y''-terms together in their own respective square terms, then we will have the equation in the standard form
As the equation stands, it is difficult directly to know anything about the circle, but if we complete the square and combine ''x''- and ''y''-terms together in their own respective square terms, then we will have the equation in the standard form
-
{{Displayed math||<math>(x-a)^2 + (y-b)^2 = r^2\,,</math>}}
+
{{Abgesetzte Formel||<math>(x-a)^2 + (y-b)^2 = r^2\,,</math>}}
and we will then be able to read off the circle's centre and radius.
and we will then be able to read off the circle's centre and radius.
Zeile 7: Zeile 7:
If we take the ''x''- and ''y''-terms on the left-hand side and complete the square, we get
If we take the ''x''- and ''y''-terms on the left-hand side and complete the square, we get
-
{{Displayed math||<math>\begin{align}
+
{{Abgesetzte Formel||<math>\begin{align}
x^2 + 2x &= (x+1)^2-1^2\,,\\[5pt]
x^2 + 2x &= (x+1)^2-1^2\,,\\[5pt]
y^2 - 2y &= (y-1)^2-1^2\,,
y^2 - 2y &= (y-1)^2-1^2\,,
Zeile 14: Zeile 14:
and then the whole equation can be written as
and then the whole equation can be written as
-
{{Displayed math||<math>(x+1)^2 - 1^2 + (y-1)^2 - 1^2 = 1\,,</math>}}
+
{{Abgesetzte Formel||<math>(x+1)^2 - 1^2 + (y-1)^2 - 1^2 = 1\,,</math>}}
or, with the constants moved to the right-hand side,
or, with the constants moved to the right-hand side,
-
{{Displayed math||<math>(x+1)^2 + (y-1)^2 = 3\,\textrm{.}</math>}}
+
{{Abgesetzte Formel||<math>(x+1)^2 + (y-1)^2 = 3\,\textrm{.}</math>}}
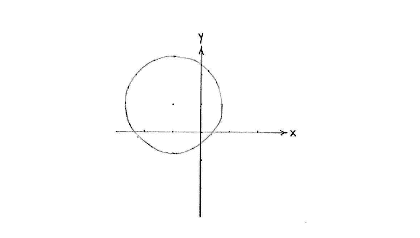
This is a circle having its centre at (-1,1) and radius <math>\sqrt{3}\,</math>.
This is a circle having its centre at (-1,1) and radius <math>\sqrt{3}\,</math>.

Version vom 08:49, 22. Okt. 2008

As the equation stands, it is difficult directly to know anything about the circle, but if we complete the square and combine x- and y-terms together in their own respective square terms, then we will have the equation in the standard form

\displaystyle (x-a)^2 + (y-b)^2 = r^2\,,

and we will then be able to read off the circle's centre and radius.

If we take the x- and y-terms on the left-hand side and complete the square, we get

\displaystyle \begin{align}

x^2 + 2x &= (x+1)^2-1^2\,,\\[5pt] y^2 - 2y &= (y-1)^2-1^2\,, \end{align}

and then the whole equation can be written as

\displaystyle (x+1)^2 - 1^2 + (y-1)^2 - 1^2 = 1\,,

or, with the constants moved to the right-hand side,

\displaystyle (x+1)^2 + (y-1)^2 = 3\,\textrm{.}

This is a circle having its centre at (-1,1) and radius \displaystyle \sqrt{3}\,.


Image:4_1_7a-2(2).gif
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.1:7a“