Lösung 4.4:1f
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
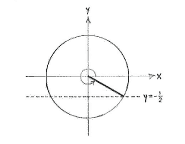
| - | We can translate the equation | + | We can translate the equation <math>\sin v=-1/2</math> to the problem of finding those angles in the unit circle which have a ''y''-coordinate of <math>-1/2</math>. If we compare this with the problem that we had in exercise a, where we looked for angles which satisfied <math>\sin v = +1/2</math>, then the situation is the same, except that the angles now lie under, rather than above, the ''x''-axis, due to reflectional symmetry. |
| - | <math>\sin v=- | + | |
| - | to the problem of finding those angles in the unit circle which have a y-coordinate of | + | |
| - | <math>- | + | |
| - | <math>\sin v=+ | + | |
| - | + | ||
| - | -axis, due to reflectional symmetry. | + | |
| + | {| align="center" | ||
| + | | align="center" |[[Image:4_4_1_f-1.gif|center]] | ||
| + | | width="20px" | | ||
| + | | align="center" |[[Image:4_4_1_f-2.gif|center]] | ||
| + | |- | ||
| + | | align="center" |<small>Angle 2π - π/6 = 11π/6</small> | ||
| + | || | ||
| + | | align="center" |<small>Angle π + π/6 = 7π/6</small> | ||
| + | |} | ||
| - | + | The two angles which satisfy <math>\sin v=-1/2</math> lie in the third and fourth quadrants and are <math>v=2\pi - \pi/6 = 11\pi/6</math> and <math>v = \pi + \pi/6 = 7\pi/6</math>. | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | The two angles which satisfy | + | |
| - | <math>\sin v=- | + | |
| - | lie in the third and fourth quadrants and are | + | |
| - | <math>v=2\pi - | + | |
| - | and | + | |
| - | <math>v=\pi + | + | |
Version vom 13:41, 10. Okt. 2008
We can translate the equation \displaystyle \sin v=-1/2 to the problem of finding those angles in the unit circle which have a y-coordinate of \displaystyle -1/2. If we compare this with the problem that we had in exercise a, where we looked for angles which satisfied \displaystyle \sin v = +1/2, then the situation is the same, except that the angles now lie under, rather than above, the x-axis, due to reflectional symmetry.
| Angle 2π - π/6 = 11π/6 | Angle π + π/6 = 7π/6 |
The two angles which satisfy \displaystyle \sin v=-1/2 lie in the third and fourth quadrants and are \displaystyle v=2\pi - \pi/6 = 11\pi/6 and \displaystyle v = \pi + \pi/6 = 7\pi/6.