Lösung 4.4:1a
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
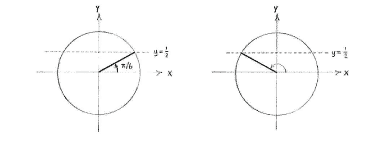
| - | In the unit circle's first quadrant, there is one angle whose sine value equals | + | In the unit circle's first quadrant, there is one angle whose sine value equals 1/2 and that is <math>v = \pi/6\,</math>. |
| - | + | ||
| - | and that is | + | |
| - | <math>v= | + | |
[[Image:4_4_1_a.gif|center]] | [[Image:4_4_1_a.gif|center]] | ||
| - | From the figures, we see that there is a further angle with the same sine value and it lies in the second quadrant. Because of symmetry, it makes the same angle with the negative | + | From the figures, we see that there is a further angle with the same sine value and it lies in the second quadrant. Because of symmetry, it makes the same angle with the negative ''x''-axis as <math>v=\pi/6</math> makes with the positive ''x''-axis, i.e. the other angle is <math>v = \pi - \pi/6 = 5\pi/6\,</math>. |
| - | + | ||
| - | -axis as | + | |
| - | <math>v= | + | |
| - | makes with the positive | + | |
| - | + | ||
| - | -axis, i.e. the other angle is | + | |
| - | <math>v=\pi - | + | |
Version vom 13:10, 10. Okt. 2008
In the unit circle's first quadrant, there is one angle whose sine value equals 1/2 and that is \displaystyle v = \pi/6\,.
From the figures, we see that there is a further angle with the same sine value and it lies in the second quadrant. Because of symmetry, it makes the same angle with the negative x-axis as \displaystyle v=\pi/6 makes with the positive x-axis, i.e. the other angle is \displaystyle v = \pi - \pi/6 = 5\pi/6\,.