Lösung 4.3:3d
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | The expression for the angle | + | The expression for the angle <math>\pi/2 - v</math> differs from <math>\pi/2</math> by as much as <math>-v</math> differs from <math>0</math>. This means that <math>\pi/2-v</math> makes the same angle with the positive ''y''-axis as <math>-v</math> makes with the positive ''x''-axis. |
| - | <math> | + | |
| - | differs from | + | |
| - | <math> | + | |
| - | by as much as | + | |
| - | <math>-v | + | |
| - | differs from | + | |
| - | <math>0</math>. This means that | + | |
| - | <math> | + | |
| - | makes the same angle with the positive | + | |
| - | + | ||
| - | -axis as | + | |
| - | <math>-v | + | |
| - | makes with the positive | + | |
| - | + | ||
| - | -axis. | + | |
| + | {| align="center" | ||
| + | | align="center" |[[Image:4_3_3_d-1.gif]] | ||
| + | | width="20px"| | ||
| + | | align="center" |[[Image:4_3_3_d-2.gif]] | ||
| + | |- | ||
| + | | align="center" |<small>Angle ''v''</small> | ||
| + | || | ||
| + | | align="center" |<small>Angle π/2 - ''v''</small> | ||
| + | |} | ||
| - | + | Therefore, the angle <math>\pi/2 - v</math> has a ''y''-coordinate which is equal to the ''x''-coordinate for the angle ''v'', i.e. | |
| - | + | {{Displayed math||<math>\sin\Bigl(\frac{\pi}{2} - v\Bigr) = \cos v</math>}} | |
| - | + | ||
| - | + | ||
| - | <math>\pi -v</math> | + | |
| - | + | ||
| - | + | and from exercise c, we know that <math>\cos v = \sqrt{1-a^2}\,</math>, | |
| - | <math> | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | {{Displayed math||<math>\sin\Bigl(\frac{\pi}{2}-v\Bigr) = \sqrt{1-a^2}\,\textrm{.}</math>}} | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | <math>\sin \ | + | |
Version vom 13:44, 9. Okt. 2008
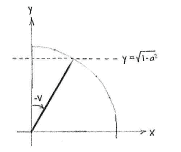
The expression for the angle \displaystyle \pi/2 - v differs from \displaystyle \pi/2 by as much as \displaystyle -v differs from \displaystyle 0. This means that \displaystyle \pi/2-v makes the same angle with the positive y-axis as \displaystyle -v makes with the positive x-axis.
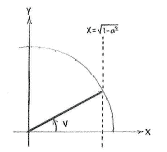
| 
| |
| Angle v | Angle π/2 - v |
Therefore, the angle \displaystyle \pi/2 - v has a y-coordinate which is equal to the x-coordinate for the angle v, i.e.
and from exercise c, we know that \displaystyle \cos v = \sqrt{1-a^2}\,,
