Lösung 4.2:8
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
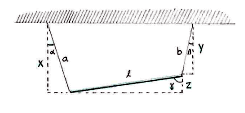
| - | We start by drawing three auxiliary triangles, and calling the three vertical sides | + | We start by drawing three auxiliary triangles, and calling the three vertical sides ''x'', ''y'' and ''z'', as shown in the figure. |
| - | + | ||
| - | and | + | |
| - | + | ||
| - | + | ||
[[Image:4_2_8.gif|center]] | [[Image:4_2_8.gif|center]] | ||
| - | Using the definition of cosine, we can work out | + | Using the definition of cosine, we can work out ''x'' and ''y'' from |
| - | + | ||
| - | and | + | |
| - | + | ||
| - | from | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>\begin{align} | ||
| + | x &= a\cos \alpha\,,\\[3pt] | ||
| + | y &= b\cos \beta\,, | ||
| + | \end{align}</math>}} | ||
| - | + | and, for the same reason, we know that ''z'' satisfies the relation | |
| + | {{Displayed math||<math>z=\ell\cos \gamma\,\textrm{.}</math>}} | ||
| - | + | In addition, we know that the lengths ''x'', ''y'' and ''z'' satisfy the equality | |
| - | + | ||
| - | and | + | |
| - | + | ||
| + | {{Displayed math||<math>z=x-y\,\textrm{.}</math>}} | ||
| - | + | If we substitute in the expressions for ''x'', ''y'' and ''z'', we obtain the trigonometric equation | |
| + | {{Displayed math||<math>\ell\cos \gamma = a\cos \alpha -b\cos \beta\,\textrm{,}</math>}} | ||
| - | where | + | where <math>\gamma </math> is the only unknown. |
| - | <math>\gamma </math> | + | |
| - | is the only unknown. | + | |
Version vom 12:23, 9. Okt. 2008
We start by drawing three auxiliary triangles, and calling the three vertical sides x, y and z, as shown in the figure.
Using the definition of cosine, we can work out x and y from
and, for the same reason, we know that z satisfies the relation
In addition, we know that the lengths x, y and z satisfy the equality
If we substitute in the expressions for x, y and z, we obtain the trigonometric equation
where \displaystyle \gamma is the only unknown.