Lösung 4.2:2f
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
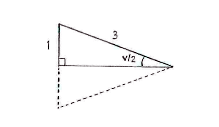
| + | Because the triangle is isosceles (two sides have the same length), it can be divided up into two right-angled triangles of the same size by introducing a side which divides the angle ''v'' in half. | ||
| + | |||
[[Image:4_2_2_f.gif|center]] | [[Image:4_2_2_f.gif|center]] | ||
| - | |||
| - | Because the triangle is isosceles (two sides have the same length), it can be divided up into two right-angled triangles of the same size by introducing a side which divides the angle | ||
| - | <math>v</math> | ||
| - | in half. | ||
If we look at one of the triangles, we can set up the trigonometrical relation | If we look at one of the triangles, we can set up the trigonometrical relation | ||
| + | {{Displayed math||<math>\sin\frac{v}{2} = \frac{1}{3}\,,</math>}} | ||
| - | + | which is an equation for ''v''. | |
| - | + | ||
| - | which is an equation for | + | |
| - | + | ||
Version vom 07:36, 9. Okt. 2008
Because the triangle is isosceles (two sides have the same length), it can be divided up into two right-angled triangles of the same size by introducing a side which divides the angle v in half.
If we look at one of the triangles, we can set up the trigonometrical relation
which is an equation for v.