Lösung 4.1:5b

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Zeile 1: Zeile 1:
-
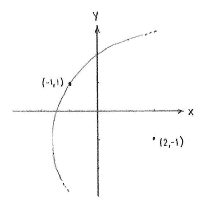
If the circle is to contain the point
+
If the circle is to contain the point (-1,1), then that point's distance away from the centre (2,-1) must equal the circle's radius, ''r''. Thus, we can obtain the circle's radius by calculating the distance between (-1,1) and (2,-1) using the distance formula,
-
<math>\left( -1 \right.,\left. 1 \right)</math>, then that point's distance away from the centre
+
-
<math>\left( 2 \right.,\left. -1 \right)</math>
+
-
must equal the circle's radius,
+
-
<math>r</math>. Thus, we can obtain the circle's radius by calculating the distance between
+
-
<math>\left( -1 \right.,\left. 1 \right)</math>
+
-
and
+
-
<math>\left( 2 \right.,\left. -1 \right)</math>
+
-
using the distance formula:
+
-
 
+
-
 
+
-
<math>\begin{align}
+
-
& r=\sqrt{\left( 2-\left( -1 \right) \right)^{2}+\left( -1-1 \right)^{2}}=\sqrt{3^{2}+\left( -2 \right)^{2}} \\
+
-
& =\sqrt{9+4}=\sqrt{13} \\
+
-
\end{align}</math>
+
 +
{{Displayed math||<math>\begin{align}
 +
r &= \sqrt{(2-(-1))^2+(-1-1)^2} = \sqrt{3^2+(-2)^2} = \sqrt{9+4} = \sqrt{13}\,\textrm{.}
 +
\end{align}</math>}}
When we know the circle's centre and its radius, we can write the equation of the circle,
When we know the circle's centre and its radius, we can write the equation of the circle,
-
 
+
{{Displayed math||<math>(x-2)^2 + (y-(-1))^2 = (\sqrt{13})^{2}</math>}}
-
<math>\left( x-2 \right)^{2}+\left( y-\left( -1 \right) \right)^{2}=\left( \sqrt{13} \right)^{2}</math>
+
-
 
+
which the same as
which the same as
 +
{{Displayed math||<math>(x-2)^{2} + (y+1)^2 = 13\,\textrm{.}</math>}}
-
<math>\left( x-2 \right)^{2}+\left( y+1 \right)^{2}=13</math>
 
-
{{NAVCONTENT_START}}
 
[[Image:4_1_5_b-1(2).gif|center]]
[[Image:4_1_5_b-1(2).gif|center]]
-
{{NAVCONTENT_STOP}}
 
- 
-
NOTE: A circle having its centre at
 
-
<math>\left( a \right.,\left. b \right)</math>
 
-
and radius
 
-
<math>r</math>
 
-
has the equation
 
 +
Note: A circle having its centre at (''a'',''b'') and radius ''r'' has the equation
-
<math>\left( x-a \right)^{2}+\left( y-b \right)2=r^{2}</math>
+
{{Displayed math||<math>(x-a)^2 + (y-b)^2 = r^2\,\textrm{.}</math>}}

Version vom 10:53, 7. Okt. 2008

If the circle is to contain the point (-1,1), then that point's distance away from the centre (2,-1) must equal the circle's radius, r. Thus, we can obtain the circle's radius by calculating the distance between (-1,1) and (2,-1) using the distance formula,

Vorlage:Displayed math

When we know the circle's centre and its radius, we can write the equation of the circle,

Vorlage:Displayed math

which the same as

Vorlage:Displayed math



Note: A circle having its centre at (a,b) and radius r has the equation

Vorlage:Displayed math

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.1:5b“