Lösung 2.3:6a
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | Using the | + | Using the rule <math>(a+b)^2=a^2+2ab+b^2</math>, we recognize the polynomial as the expansion of <math>(x-1)^{2}\,</math>, |
| - | <math> | + | |
| + | {{Displayed math||<math>x^{2}-2x+1 = (x-1)^{2}\,\textrm{.}</math>}} | ||
| - | <math>x | + | This quadratic expression has its smallest value, zero, when <math>x-1=0</math>, i.e. |
| + | <math>x=1</math>. All non-zero values of <math>x-1</math> give a positive value for | ||
| + | <math>(x-1)^{2}</math>. | ||
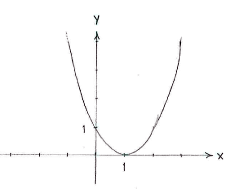
| - | + | Note: If we draw the curve <math>y=(x-1)^{2}</math>, we see that it has a minimum value of zero at <math>x=1\,</math>. | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | <math>y= | + | |
| - | <math>x=\ | + | |
[[Image:2_3_6_a.gif|center]] | [[Image:2_3_6_a.gif|center]] | ||
Version vom 11:29, 29. Sep. 2008
Using the rule \displaystyle (a+b)^2=a^2+2ab+b^2, we recognize the polynomial as the expansion of \displaystyle (x-1)^{2}\,,
This quadratic expression has its smallest value, zero, when \displaystyle x-1=0, i.e. \displaystyle x=1. All non-zero values of \displaystyle x-1 give a positive value for \displaystyle (x-1)^{2}.
Note: If we draw the curve \displaystyle y=(x-1)^{2}, we see that it has a minimum value of zero at \displaystyle x=1\,.