Aus Online Mathematik Brückenkurs 1

Version vom 09:02, 18. Sep. 2008 (bearbeiten) Ian (Diskussion | Beiträge) ← Zum vorherigen Versionsunterschied		Version vom 11:55, 24. Sep. 2008 (bearbeiten) (rückgängig) Tek (Diskussion | Beiträge) K Zum nächsten Versionsunterschied →
Zeile 1:		Zeile 1:
	Let's write down the equation for a straight line as		Let's write down the equation for a straight line as
		+	{{Displayed math||<math>y=kx+m\,,</math>}}
-	<math>y=kx+m</math>	+	where ''k'' and ''m'' are constants which we shall determine.
		+	Since the points (2,3) and (3,0) should lie on the line, they must also satisfy the equation of the line,
-	where	+	{{Displayed math||<math>3=k\cdot 2+m\qquad\text{and}\qquad 0=k\cdot 3+m\,\textrm{.}</math>}}
-	<math>k</math>	+
-	and	+
-	<math>m</math>	+
-	are constants which we shall determine.	+
-	Since the points	+	If we take the difference between the equations, ''m'' disappears and we can work out the slope ''k'',
-	<math>\left( 2 \right., \left. 3 \right)</math>	+
-	and	+
-	<math>\left( 3 \right., \left. 0 \right)</math>	+
-	should lie on the line, they must also satisfy the equation of the line,	+
		+	{{Displayed math||<math>\begin{align}
		+	3-0 &= k\cdot 2+m-(k\cdot 3+m)\,,\\[5pt]
		+	3 &= -k\,\textrm{.}
		+	\end{align}</math>}}
-	<math>3=k\centerdot 2+m</math>	+	Substituting this into the equation <math>0=k\centerdot 3+m</math> then gives us a value for ''m'',
-	and	+
-	<math>0=k\centerdot 3+m</math>	+
		+	{{Displayed math||<math>m=-3k=-3\cdot (-3)=9\,\textrm{.}</math>}}
-	If we take the difference between the equations,	+	The equation of the line is thus <math>y=-3x+9</math>.
-	<math>m</math>	+
-	disappears and we can work out the gradient	+
-	<math>k</math>,	+
-	<math>3-0=k\centerdot 2+m-\left( k\centerdot 3+m \right)</math>	+	<center>[[Image:S1_2_2_5_a.jpg]]</center>
-	<math>3=-k</math>	+	Note. To be completely certain that we have calculated correctly, we check that the points (2,3) and (3,0) satisfy the equation of the line:
-	Substituting this into the equation	+	:*(''x'',''y'')&nbsp;=&nbsp;(2,3): <math>\text{LHS} = 3\ </math> and <math>\ \text{RHS} = -3\cdot 2+9 = 3\,</math>.
-	<math>0=k\centerdot 3+m</math>	+	:*(''x'',''y'')&nbsp;=&nbsp;(3,0): <math>\text{LHS} = 0\ </math> and <math>\ \text{LHS} = -3\cdot 3+9 = 0\,</math>.
-	then gives us a value for	+
-	<math>m</math>,	+
-		+
-		+
-	<math>m=-3k=-3\centerdot \left( -3 \right)=9</math>	+
-		+
-		+
-	The equation of the line is thus	+
-	<math>y=-3x+9</math>.	+
-		+
-		+
-		+
-	NOTE: To be completely certain that we have calculated correctly, we check that the points	+
-	<math>\left( 2 \right., \left. 3 \right)</math>	+
-	and	+
-	<math>\left( 3 \right., \left. 0 \right)</math>	+
-	satisfy the equation of the line:	+
-		+
-	<math>\left( x \right., \left. y \right)=\left( 2 \right., \left. 3 \right)</math>: LHS=	+
-	<math>3</math>	+
-	and RHS=	+
-	<math>-3\centerdot 2+9=3</math>	+
-		+
-		+
-	<math>\left( x \right., \left. y \right)=\left( 3 \right., \left. 0 \right)</math>: LHS=	+
-	<math>0</math>	+
-	and LHS=	+
-	<math>-3\centerdot 3+9=0</math>	+
-		+
-		+
-		+
-	{{NAVCONTENT_START}}	+
-	<!--<center> [[Image:2_2_5a-1(2).gif]] </center>-->	+
-	{{NAVCONTENT_STOP}}	+
-	{{NAVCONTENT_START}}	+
-		+
-	[[Image:S1_2_2_5_a.jpg]]	+
-	<!--<center> [[Image:2_2_5a-2(2).gif]] </center>-->	+
-	{{NAVCONTENT_STOP}}	+

---

Version vom 11:55, 24. Sep. 2008

Let's write down the equation for a straight line as

Vorlage:Displayed math

where k and m are constants which we shall determine.

Since the points (2,3) and (3,0) should lie on the line, they must also satisfy the equation of the line,

Vorlage:Displayed math

If we take the difference between the equations, m disappears and we can work out the slope k,

Vorlage:Displayed math

Substituting this into the equation \displaystyle 0=k\centerdot 3+m then gives us a value for m,

Vorlage:Displayed math

The equation of the line is thus \displaystyle y=-3x+9.

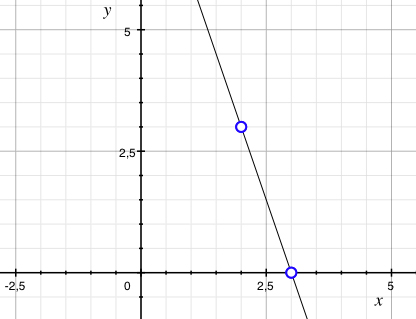
Note. To be completely certain that we have calculated correctly, we check that the points (2,3) and (3,0) satisfy the equation of the line:

• (x,y) = (2,3): \displaystyle \text{LHS} = 3\ and \displaystyle \ \text{RHS} = -3\cdot 2+9 = 3\,.
• (x,y) = (3,0): \displaystyle \text{LHS} = 0\ and \displaystyle \ \text{LHS} = -3\cdot 3+9 = 0\,.