Lösung 4.4:5a

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 4.4:5a moved to Solution 4.4:5a: Robot: moved page)
Zeile 1: Zeile 1:
-
{{NAVCONTENT_START}}
+
If we consider for a moment the equality
-
<center> [[Image:4_4_5a-1(2).gif]] </center>
+
 
-
{{NAVCONTENT_STOP}}
+
 
-
{{NAVCONTENT_START}}
+
<math>\sin u=\sin v\quad \quad \quad (*)</math>
-
<center> [[Image:4_4_5a-2(2).gif]] </center>
+
 
-
{{NAVCONTENT_STOP}}
+
 
 +
 
 +
where
 +
<math>u</math>
 +
has a fixed value, there are usually two angles
 +
<math>v</math>
 +
in the unit circle which ensure that the equality holds:
 +
 
 +
 
 +
<math>v=u</math>
 +
and
 +
<math>v=\pi -u</math>
 +
 
[[Image:4_4_5_a.gif]]
[[Image:4_4_5_a.gif]]
 +
 +
 +
(The only exception is when
 +
<math>u={\pi }/{2}\;\text{ }</math>
 +
or
 +
<math>u=3{\pi }/{2}\;\text{ }</math>, in which case
 +
<math>u</math>
 +
and
 +
<math>\pi -u\text{ }</math>
 +
correspond to the same direction and there is only one angle
 +
<math>v</math>
 +
which satisfies the equality.)
 +
 +
We obtain all the angles
 +
<math>v</math>
 +
which satisfy (*) by adding multiples of
 +
<math>\text{2}\pi </math>,
 +
 +
 +
<math>v=u+2n\pi </math>
 +
and
 +
<math>v=\pi -u+2n\pi </math>
 +
 +
 +
where
 +
<math>n\text{ }</math>
 +
is an arbitrary integer.
 +
 +
If we now go back to our equation
 +
 +
 +
<math>\sin 3x=\sin x</math>
 +
 +
 +
the reasoning above shows that the equation is only satisfied when
 +
 +
 +
<math>3x=x+2n\pi </math>
 +
or
 +
<math>3x=\pi -x+2n\pi </math>
 +
 +
 +
If we make
 +
<math>x</math>
 +
the subject of each equation, we obtain the full solution to the equation:
 +
 +
 +
<math>\left\{ \begin{array}{*{35}l}
 +
x=0+n\pi \\
 +
x=\frac{\pi }{4}+\frac{1}{2}n\pi \\
 +
\end{array} \right.</math>

Version vom 10:55, 1. Okt. 2008

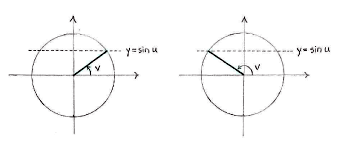
If we consider for a moment the equality


\displaystyle \sin u=\sin v\quad \quad \quad (*)


where \displaystyle u has a fixed value, there are usually two angles \displaystyle v in the unit circle which ensure that the equality holds:


\displaystyle v=u and \displaystyle v=\pi -u


Image:4_4_5_a.gif


(The only exception is when \displaystyle u={\pi }/{2}\;\text{ } or \displaystyle u=3{\pi }/{2}\;\text{ }, in which case \displaystyle u and \displaystyle \pi -u\text{ } correspond to the same direction and there is only one angle \displaystyle v which satisfies the equality.)

We obtain all the angles \displaystyle v which satisfy (*) by adding multiples of \displaystyle \text{2}\pi ,


\displaystyle v=u+2n\pi and \displaystyle v=\pi -u+2n\pi


where \displaystyle n\text{ } is an arbitrary integer.

If we now go back to our equation


\displaystyle \sin 3x=\sin x


the reasoning above shows that the equation is only satisfied when


\displaystyle 3x=x+2n\pi or \displaystyle 3x=\pi -x+2n\pi


If we make \displaystyle x the subject of each equation, we obtain the full solution to the equation:


\displaystyle \left\{ \begin{array}{*{35}l} x=0+n\pi \\ x=\frac{\pi }{4}+\frac{1}{2}n\pi \\ \end{array} \right.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.4:5a“