Processing Math: Done
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

Lösung 4.1:7d

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 4.1:7d moved to Solution 4.1:7d: Robot: moved page)
Zeile 1: Zeile 1:
 +
We rewrite the equation in standard by completing the square for the x- and y-terms:
 +
 +
 +
<math>x^{2}-2x=\left( x-1 \right)^{2}-1^{2}</math>
 +
 +
 +
<math>y^{2}+2y=\left( y+1 \right)^{2}-1^{2}</math>
 +
 +
 +
Now, the equation is
 +
 +
 +
<math>\begin{align}
 +
& \left( x-1 \right)^{2}-1+\left( y+1 \right)^{2}-1=-2 \\
 +
& \Leftrightarrow \quad \left( x-1 \right)^{2}+\left( y+1 \right)^{2}=0 \\
 +
\end{align}</math>
 +
 +
 +
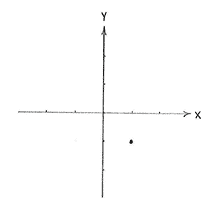
The only point which satisfies this equation is
 +
<math>\left( x \right.,\left. y \right)=\left( 1 \right.,\left. -1 \right)</math>
 +
because, for all other values of
 +
<math>x</math>
 +
and
 +
<math>y</math> , the left-hand side is strictly positive and therefore not zero.
 +
 +
{{NAVCONTENT_START}}
{{NAVCONTENT_START}}
<center> [[Image:4_1_7_d.gif]] </center>
<center> [[Image:4_1_7_d.gif]] </center>
-
<center> [[Image:4_1_7d.gif]] </center>
+
 
{{NAVCONTENT_STOP}}
{{NAVCONTENT_STOP}}

Version vom 12:12, 27. Sep. 2008

We rewrite the equation in standard by completing the square for the x- and y-terms:


x2−2x=x−12−12 


y2+2y=y+12−12 


Now, the equation is


x−12−1+y+12−1=−2x−12+y+12=0


The only point which satisfies this equation is xy=1−1  because, for all other values of x and y , the left-hand side is strictly positive and therefore not zero.


Image:4_1_7_d.gif


Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.1:7d“